Rhombus

Rhombus
Rhombus
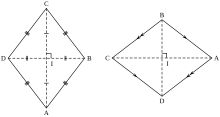	A rhombus in two different orientations
Type	quadrilateral, trapezoid, parallelogram, kite
Edges and vertices	4
Schläfli symbol	{ } + { }; {2α}
Coxeter–Dynkin diagrams
Symmetry group	Dihedral (D2), [2], (*22), order 4
Area	(half the product of the diagonals)
Properties	convex, isotoxal

평면 유클리드 기하학(Euclidean geometry)에서, 마름모(rhombus, 복수형 rhombi 또는 rhombuses)는 그것의 네 변 모두가 같은 길이를 가지는 사변형(quadrilateral)입니다. 또 다른 이름은 등변 사변형(equilateral quadrilateral)인데, 왜냐하면 등변은 그것의 모든 변이 길이에서 같음을 의미하기 때문입니다. 모름모는 종종 팔면체(octahedral) 다이아몬드(diamond), 또는 마름모꼴(lozenge)의 투영과 닮은 플레잉 카드(playing card)에서 다이아몬드(diamonds) 벌을 따서 다이아몬드(diamond)라고 불리지만, 전자는 때때로 60° 각도를 갖는 마름모를 구체적으로 특별히 참조하고 (이것을 일부 저자는 French sweet를 따서 calisson으로 불립니다^[1] – 역시 폴리아몬드(Polyiamond)를 참조하십시오), 후자는 때때로 45° 각도를 갖는 마름모를 구체적으로 특별히 참조합니다.

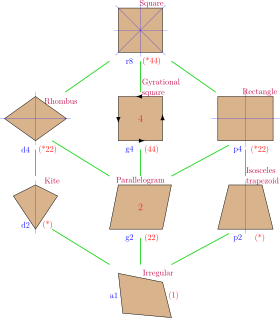
모든 각 마름모는 단순(simple) (비-자체-교차하는 것)이고, 평행사변형(parallelogram)과 연(kite)의 특수한 경우입니다. 직각을 갖는 마름모는 정사각형(square)입니다.^[2]^[3]

Etymology

단어 "마름모"는 Ancient Greek: ῥόμβος, romanized: rhombos에서 파생되며, 회전하는 어떤 것을 의미하며,^[4] 이것은 동사 ῥέμβω, romanized: rhémbō에서 파생되며, "빙글 빙글 회전하는 것"을 의미합니다.^[5] 그 단어는 유클리드(Euclid)와 아르키메데스(Archimedes) 둘 다에 의해 사용되었으며, 그는 공통 밑변을 공유하는 둘의 직각 원형 원뿔(cone)인 이중-원뿔(bicone)에 대해 용어 "고체 마름모"를 사용했습니다.^[6]

오늘날 우리가 rhombus라고 참조하는 표면은 평면 위에 둘의 원뿔의 꼭대기를 통과하는 이중-원뿔의 교차 단면(cross section)입니다.

Characterizations

단순(simple) (비-자체-교차하는(self-intersecting)) 사변형이 마름모인 것과 그것이 다음 중 임의의 하나인 것은 필요충분(iff) 조건입니다:^[7]^[8]

대각선(diagonal)이 내부 각도(interior angle)를 이등분하는 평행사변형(parallelogram)
적어도 둘의 연속적인 변이 길이에서 같은 평행사변형
대각선이 수직인 평행사변형 (직교대각선(orthodiagonal) 평행사변형)
(정의에 의해) 같은 길이의 넷의 변을 갖는 사변형
대각선이 수직(perpendicular)이고 서로를 이등분(bisect)하는 사변형
대각선이 둘의 반대편 내부 각도를 이등분하는 사변형
넷의 삼각형 ABP, BCP, CDP, 및 DAP가 모두 합동(congruent)을 만족하는 그것의 평면에서 점 P를 소유하는 사변형 ABCD^[9]
삼각형 ABC, BCD, CDA, 및 DAB에서 내원(incircle)이 하나의 공통 점을 가지는 사변형 ABCD^[10]

Basic properties

모든 각 마름모는 반대편 꼭짓점의 쌍을 연결하는 둘의 대각선(diagonal)과 두 쌍의 평행한 변을 가집니다. 합동(congruent) 삼각형(triangle)을 사용하여, 우리는 마름모가 각 이들 대각선에 걸쳐 대칭(symmetric)임을 입증(prove)할 수 있습니다. 임의의 마름모는 다음 속성을 가짐을 따릅니다:

마름모의 반대편 각도(angle)는 같은 측정을 가집니다.
마름모의 둘의 대각선은 수직(perpendicular)입니다; 즉, 마름모는 직교대각선 사변형(orthodiagonal quadrilateral)입니다.
그것의 대각선은 반대편 각도를 이등분합니다.

첫 번째 속성은 모든 각 마름모가 평행사변형(parallelogram)임을 의미합니다. 마름모는 따라서 평행사변형의 모든 속성을 가집니다: 예를 들어, 반대쪽 변은 평행합니다; 인접한 각도는 보충적(supplementary)입니다; 두 대각선은 서로를 이등분(bisect)합니다; 중간점을 통과하는 임의의 직선은 넓이를 이등분합니다; 그리고 변의 제곱의 합은 대각선의 제곱의 합과 같습니다 (평행사변형 법칙(parallelogram law)). 따라서 모든 각 마름모에서, 공통 변을 a를 나타내고 p와 q로 대각선을 나타내면,

\displaystyle 4a^{2}=p^{2}+q^{2}.

모든 각 평행사변형이 마름모는 아니지만, 수직 대각선 (두 번째 속성)을 갖는 평행사변형은 마름모입니다. 일반적으로, 대각선 중 하나가 대칭의 직선인 수직 대각선을 갖는 임의의 사각형은 연(kite)입니다. 모든 각 마름모는 연이고, 연과 평행사변형 둘 다인 임의의 사변형은 마름모입니다.

마름모는 접하는 사변형(tangential quadrilateral)입니다.^[11] 즉, 그것은 모든 네 변에 접하는 내접된 원(inscribed circle)을 가집니다.

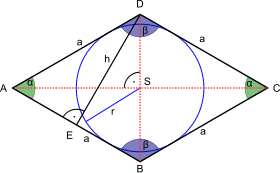
A rhombus. Each angle marked with a black dot is a right angle. The height h is the perpendicular distance between any two non-adjacent sides, which equals the diameter of the circle inscribed. The diagonals of lengths p and q are the red dotted line segments.

Diagonals

대각선의 길이 p = AC와 q = BD는 마름모 변 a와 하나의 꼭짓점 각도 α의 관점에서 다음으로 표현될 수 있습니다:

p=a{\sqrt {2+2\cos {\alpha }}}

및

q=a{\sqrt {2-2\cos {\alpha }}}.

이들 공식은 코사인의 법칙(law of cosines)의 직접 결과입니다.

Inradius

내반지름 (마름모에 내접된(inscribed) 원의 반지름)은, $r$ 에 의해 표시되며, 대각선 $p$ 와 $q$ 의 관점에서 다음으로 표현될 수 있습니다:^[11]

r={\frac {p\cdot q}{2{\sqrt {p^{2}+q^{2}}}}},

또는 변 길이 $a$ 와 임의의 꼭짓점 각도 $α$ 또는 $β$ 의 관점에서 다음으로 표현될 수 있습니다:

r={\frac {a\sin \alpha }{2}}={\frac {a\sin \beta }{2}}.

Area

모든 평행사변형(parallelogram)에 대한 것처럼, 마름모의 넓이(area) K는 그것의 밑변(base)과 그것의 높이(height) (h)의 곱입니다. 밑변은 단순한 임의의 변 길이 a입니다:

K=a\cdot h.

그 넓이는 역시 제곱된(squared) 밑변(base) 곱하기 임의의 각도의 사인으로 표현될 수 있습니다:

K=a^{2}\cdot \sin \alpha =a^{2}\cdot \sin \beta ,

또는 높이와 꼭짓점(vertex) 각도(angle)의 항으로 표현될 수 있습니다:

K={\frac {h^{2}}{\sin \alpha }},

또는 대각선(diagonal) p, q의 곱의 절반으로,

K={\frac {p\cdot q}{2}},

또는 반둘레(semiperimeter) 곱하기 마름모에 내접된(inscribed) 원(circle)의 반지름(radius) (내반지름)으로,

K=2a\cdot r.

평행 사변형과 공통으로, 또 다른 방법은 둘의 인접한 변을 벡터로 고려하는 것으로, 이중벡터(bivector)를 형성하므로, 넓이는 이중벡터의 크기 (두 벡터의 벡터 곱의 크기)이며, 이것은 두 벡터의 데카르트 좌표의 행렬식(determinant): K = x₁y₂ – x₂y₁입니다.^[12]

Dual properties

마름모의 이중 다각형(dual polygon)은 직사각형(rectangle)입니다:^[13]

마름모는 모두 같은 변을 가지지만, 직사각형은 모든 같은 각도를 가집니다.
마름모는 같은 반대편 각도를 가지지만, 직사각형은 같은 반대편 각도를 가집니다.
마름모는 내접된 원을 가지지만, 직사각형은 둘레원(circumcircle)을 가집니다.
마름모는 각 쌍의 반대편 꼭짓점 각도를 통해 대칭의 축을 갖지지만, 직사각형은 각 쌍의 반대편 변을 통해 대칭의 축을 가집니다.
마름모의 대각선은 같은 각도에서 교차하지만, 직사각형의 대각선은 길이에서 같습니다.
마름모의 변의 중간점을 연결함으로써 형성된 도형은 직사각형(rectangle)이고, 그 반대의 경우도 마찬가지입니다.

Cartesian equation

원점을 중심으로 하는 마름모의 변은 다음을 만족시키는 모든 점 (x, y)로 구성되는 축 위에 각각 떨어지는 대각선을 가집니다:

\left|{\frac {x}{a}}\right|\!+\left|{\frac {y}{b}}\right|\!=1.

꼭짓점은 $(\pm a,0)$ 와 $(0,\pm b)$ 에 있습니다. 이것은 지수 1을 갖는 초-타원(superellipse)의 특별한 경우입니다.

Other properties

다섯 2D 격자(lattice) 유형 중 하나는 역시 중심 직사각형 격자(centered rectangular lattice)라고 불리는 마름모꼴 격자입니다.
동일한 마름모는 60° 마름모에 대해, 마름모꼴 타일링(rhombille tiling)을 포함하여 셋의 다른 방법으로 2D 평면을 타일링할 수 있습니다.

As topological square tilings		As 30-60 degree rhombille tiling

마름모의 삼-차원 유사체에는 쌍-각뿔(bipyramid)과 이중-원뿔(bicone)을 포함합니다.
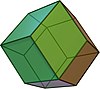
여러 다면체(polyhedra)는 마름모꼴 십이면체(rhombic dodecahedron)과 사다리-마름모꼴 십이면체(trapezo-rhombic dodecahedron)와 같은 마름모꼴 면을 가집니다.

Some polyhedra with all rhombic faces
Isohedral polyhedra		Not isohedral polyhedra
Identical rhombi	Identical golden rhombi		Two types of rhombi	Three types of rhombi

Rhombic dodecahedron	Rhombic triacontahedron	Rhombic icosahedron	Rhombic enneacontahedron	Rhombohedron

As the faces of a polyhedron

마름모면체 (역시 마름모꼴 육면체라고 불림)은 세 쌍의 평행 면이 직사각형 대신 최대 3 마름모의 유형을 제외하고는 직육면체(cuboid) (역시 직사각형 평행면체라고 불림)와 같은 삼-차원 도형입니다.

마름모꼴 십이면체(rhombic dodecahedron)는 12 합동(congruent) 마름모를 그것의 면(faces)으로 갖는 볼록 다면체(convex polyhedron)입니다.

마름모 삼각면체(rhombic triacontahedron)는 30 황금 마름모(golden rhombi) (그것의 대각선이 황금 비율(golden ratio)에 있는 마름모)를 그것의 면으로 갖는 볼록 다면체(convex polyhedron)입니다.

큰 마름모 삼각면체(great rhombic triacontahedron)는 30 교차하는 마름모 면을 갖는 비-볼록 이십면체(isohedral), 등변형(isotoxal) 다면체(polyhedron)입니다.

마름모꼴 육십면체(rhombic hexecontahedron)는 마름모꼴 삼각면체의 별모양화(stellation)입니다. 그것은 이십면체 대칭(icosahedral symmetry)을 갖는 60 황금 마름모(golden rhombic) 면을 갖는 비-볼록입니다.

마름모꼴 구십면체(rhombic enneacontahedron)는 90 마름모 면으로 구성된 다면체이며, 각 꼭짓점에서 셋, 다섯 또는 여섯 마름모가 만납니다. 그것은 60 넓은 마름모와 30 얇은 마름모를 가집니다.

사다리-마름모꼴 십이면체(trapezo-rhombic dodecahedron)는 6 마름모꼴과 6 사다리꼴(trapezoid) 면을 갖는 볼록 다면체입니다.

마름모꼴 이십면체(rhombic icosahedron)는 20 마름모꼴 면으로 구성된 다면체이며, 그 중 셋, 넷, 또는 다섯이 각 꼭짓점에서 만납니다. 그것은 적도를 따르는 10 면을 갖는 극 축 위에 10 면을 가집니다.

References

^ Alsina, Claudi; Nelsen, Roger B. (31 December 2015). A Mathematical Space Odyssey: Solid Geometry in the 21st Century. ISBN 9781614442165.
^ Note: Euclid's original definition and some English dictionaries' definition of rhombus excludes squares, but modern mathematicians prefer the inclusive definition.
^ Weisstein, Eric W. "Square". MathWorld. inclusive usage
^ ῥόμβος Archived 2013-11-08 at the Wayback Machine, Henry George Liddell, Robert Scott, A Greek-English Lexicon, on Perseus
^ ρέμβω Archived 2013-11-08 at the Wayback Machine, Henry George Liddell, Robert Scott, A Greek-English Lexicon, on Perseus
^ "The Origin of Rhombus". Archived from the original on 2015-04-02. Retrieved 2005-01-25.
^ Zalman Usiskin and Jennifer Griffin, "The Classification of Quadrilaterals. A Study of Definition Archived 2020-02-26 at the Wayback Machine", Information Age Publishing, 2008, pp. 55-56.
^ Owen Byer, Felix Lazebnik and Deirdre Smeltzer, Methods for Euclidean Geometry Archived 2019-09-01 at the Wayback Machine, Mathematical Association of America, 2010, p. 53.
^ Paris Pamfilos (2016), "A Characterization of the Rhombus", Forum Geometricorum 16, pp. 331–336, [1] Archived 2016-10-23 at the Wayback Machine
^ "IMOmath, "26-th Brazilian Mathematical Olympiad 2004"" (PDF). Archived (PDF) from the original on 2016-10-18. Retrieved 2020-01-06.
^ ^a ^b Weisstein, Eric W. "Rhombus". MathWorld.
^ WildLinAlg episode 4 Archived 2017-02-05 at the Wayback Machine, Norman J Wildberger, Univ. of New South Wales, 2010, lecture via youtube
^ de Villiers, Michael, "Equiangular cyclic and equilateral circumscribed polygons", Mathematical Gazette 95, March 2011, 102-107.

External links

Parallelogram and Rhombus - Animated course (Construction, Circumference, Area)
Rhombus definition, Math Open Reference with interactive applet.
Rhombus area, Math Open Reference - shows three different ways to compute the area of a rhombus, with interactive applet

[1] Alsina, Claudi; Nelsen, Roger B. (31 December 2015). A Mathematical Space Odyssey: Solid Geometry in the 21st Century. ISBN 9781614442165.

[2] Note: Euclid's original definition and some English dictionaries' definition of rhombus excludes squares, but modern mathematicians prefer the inclusive definition.

[3] Weisstein, Eric W. "Square". MathWorld. inclusive usage

[4] ῥόμβος Archived 2013-11-08 at the Wayback Machine, Henry George Liddell, Robert Scott, A Greek-English Lexicon, on Perseus

[5] ρέμβω Archived 2013-11-08 at the Wayback Machine, Henry George Liddell, Robert Scott, A Greek-English Lexicon, on Perseus

[6] "The Origin of Rhombus". Archived from the original on 2015-04-02. Retrieved 2005-01-25.

[7] Zalman Usiskin and Jennifer Griffin, "The Classification of Quadrilaterals. A Study of Definition Archived 2020-02-26 at the Wayback Machine", Information Age Publishing, 2008, pp. 55-56.

[8] Owen Byer, Felix Lazebnik and Deirdre Smeltzer, Methods for Euclidean Geometry Archived 2019-09-01 at the Wayback Machine, Mathematical Association of America, 2010, p. 53.

[9] Paris Pamfilos (2016), "A Characterization of the Rhombus", Forum Geometricorum 16, pp. 331–336, [1] Archived 2016-10-23 at the Wayback Machine

[10] "IMOmath, "26-th Brazilian Mathematical Olympiad 2004"" (PDF). Archived (PDF) from the original on 2016-10-18. Retrieved 2020-01-06.

[Mathworld-11] Weisstein, Eric W. "Rhombus". MathWorld.

[12] WildLinAlg episode 4 Archived 2017-02-05 at the Wayback Machine, Norman J Wildberger, Univ. of New South Wales, 2010, lecture via youtube

[13] Villiers, Michael, "Equiangular cyclic and equilateral circumscribed polygons", Mathematical Gazette 95, March 2011, 102-107.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]