Trigonometry
| Trigonometry |
|---|
 |
| Reference |
| Laws and theorems |
| Calculus |
삼각법(Trigonometry) (그리스어(Greek) trigōnon로부터, "삼각형(triangle)"과 metron로부터, "측정(measure)"[1])은 삼각형(triangle)의 변 길이와 각도(angle) 사이의 관계를 연구하는 수학(mathematics)의 한 가지입니다. 그 분야는 기하학(geometry)의 응용으로부터 천문학(Astronomy) 연구까지 기원전 3세기 헬레니즘 시대(Hellenistic world) 동안에 나타났습니다.[2] 그리스인은 현의 계산(calculation of chords)에 중점을 두었으며, 반면에 인도에서 수학자들은 사인(sine)과 같은 (역시 삼각 함수(trigonometric functions)로 불리는) 삼각법의 비율에 대해 가장-초기에-알려진 값의 테이블을 만들었습니다.[3]
역사를 통틀어, 삼각법은 측지(geodesy), 측량(surveying), 천체 역학(celestial mechanics), 및 항해술(navigation)과 같은 영역에서 적용되어 왔습니다.[4]
삼각법은 그의 많은 항등식(identities)에 대해 알려져 있으며,[5][6] 이것은 방정식을 푸는 것, 보다 유용한 표현을 찾는 것, 또는 새로운 관계를 발견하기 위한 삼각법 표현을 다시-쓰는 것에 대해 사용되는 방정식입니다.[7]
History

수메르(Sumer) 천문학자들은 360도로 원의 분할을 사용하여 각도 측정을 연구했습니다.[9] 그들, 및 나중에 바빌로니아인(Babylonians)은 닮은(similar) 삼각형의 변의 비율을 연구하고 이들 비율의 일부 속성을 발견했었지만 삼각형의 변과 각도를 찾기 위한 시스템적 방법으로 바꾸지 못했습니다. 고대 누비아인(ancient Nubians)은 비슷한 방법을 사용했습니다.[10]
기원전 3세기에서, 유클리드(Euclid) 및 아르키메데스(Archimedes)와 같은 헬레니즘 수학자들(Hellenistic mathematicians)은 원에서 내접된 각(inscribed angle)과 현(chords)의 속성과 연구했었고, 그들은, 비록 그들이 대수적이라기 보다는 기하학적으로 그것을 제시했을지라도, 현대의 삼각법 공식과 동등한 이론을 입증했습니다. 기원전 140년에서, 히파르코스(Hipparchus) (소아시아, 니케아(Nicaea) 출신)는, 현대 사인 값의 테이블과 유사한, 첫 번째 현의 테이블을 제공했고, 그들을 삼각법과 구형 삼각법(spherical trigonometry)의 문제를 풀기 위해 사용했습니다.[11] 기원후 2세기에서, 그리스-이집트 천문학자 프톨레마이오스(Ptolemy) (이집트, 알렉산드리아 출신)는 그의 Almagest의 1권, 11장 (Almagest)에서 상세한 삼각법 테이블 (현의 프톨레마이오스의 테이블(Ptolemy's table of chords))을 구성했습니다.[12] 프톨레마이오스는 그의 삼각 함수를 정의하기 위해 현(chord) 길이를 사용했으며, 우리가 오늘날 사용하는 사인(sine) 규칙으로부터 약간의 차이가 있습니다.[13] (그 값은, 우리가 sin(θ)로 기억하는, 프톨레마이오스 테이블에서 관심있는 각도의 두 배 (2θ)에 대한 현 길이를 찾고, 그런-다음 해당 값을 2로 나눔으로써 찾아질 수 있습니다.) 보다 자세한 테이블이 생성되기 전에 수 세기가 지나갔고, 프톨레마이오스의 논문은 중세 비잔틴(Byzantine), 이슬람(Islamic), 및, 나중에 서유럽 세계에서 다음 1200년 동안 천문학에서 삼각법 계산을 수행하는 것에 대해 여전히 사용되었습니다.
현대 사인파 규칙은 Surya Siddhanta에서 처음으로 입증되었고, 그 속성은 (기원후) 5세기 인도의 수학자(Indian mathematician)이자 천문학자 아리아바타(Aryabhata)에 의해 추가로 문서화되었습니다.[14] 이들 그리스와 인도의 연구는 중세 이슬람 수학자(medieval Islamic mathematicians)에 의해 번역되고 확장되었습니다. 10세기에서, 이슬람 수학자들은 모두 여섯 삼각 함수를 모두 사용했었고, 그 값을 테이블로 만들었고, 구형 기하학(spherical geometry)에서 문제에 대해 그들을 적용했습니다.[15][16] 페르시아인(Persian) 폴리매쓰(polymath) 나시르 알-딘 알-투시(Nasīr al-Dīn al-Tūsī)는 그 자신의 권리에서 수학적 분야로 삼각법의 창시자로 묘사되어 왔습니다.[17][18][19] 나시르 알-딘 알-투시(Nasīr al-Dīn al-Tūsī)는 삼각법을 천문학으로 독립적인 수학적 분야로 최초로 다루었고, 그는 구형 삼각법을 현재 형태로 발전시켰습니다.[20] 그는 구형 삼각법에서 직각 삼각형의 여섯 개의 구별되는 경우를 나열했고, 그의 On the Sector Figure에서, 그는 평면과 구형 삼각형에 대해 사인의 법칙을 설명했고, 구형 삼각형에 대해 탄젠트의 법칙(law of tangents)을 발견했고, 이들 두 법칙에 대해 증명을 제공했습니다.[21] 삼각 함수와 방법의 지식은 프톨레마이오스의 그리스어 Almagest와 마찬가지로 알-바타니(Al Battani)와 나시르 알-딘 알-투시(Nasir al-din al-Tusi)와 같은 페르시아 및 아랍 천문학자의 연구의 라틴어 번역(Latin translations)을 통해 서유럽(Western Europe)에 도달했습니다.[22] 북유럽 수학자에 의한 삼각법에 관한 가장-초기의 연구 중 하나는 15세기 독일(German) 수학자 레기오몬타누스(Regiomontanus)에 의한 De Triangulis이며, 그는 비잔틴 그리스 학자 추기경 바실리오스 베사리온(Basilios Bessarion)과 함께 몇 년간 같이 생활하면서 Almagest의 사본을 작성하도록 격려받았고, 그것을 제공했습니다.[23] 같은 시대에, 그리스에서 라틴어로 Almagest의 또 다른 번역은 크레타 섬의 트레비존의 조지(George of Trebizond)에 의해 완성되었습니다.[24] 삼각법은 16세기 북유럽에서 아직도 잘 알려지지 않았으므로 니콜라우스 코페르니쿠스(Nicolaus Copernicus)는 그의 기본 개념을 설명하기 위해 De revolutionibus orbium coelestium의 두 챕터를 제공했습니다.
항해술(navigation)의 요구에 의해 시작되고 넓은 지리적 영역의 정확한 지도에 대한 요구가 증가함에 따라, 삼각법은 수학의 주요 가지로 성장했습니다.[25] 바르톨로메오 피티쿠스(Bartholomaeus Pitiscus)는 1595년에서 그의 Trigonometria를 출판하면서, 그 단어를 사용한 것이 처음이었습니다.[26] 셈마 프리시우스(Gemma Frisius)는 측량에서 오늘날 여전히 사용되는 삼각분할(triangulation)의 방법에 대해 처음으로 설명했습니다. 복소수(complex number)를 삼각법에 완전히 통합한 사람은 레온하르트 오일러(Leonhard Euler)였습니다. 17세기에서 스코틀랜드 수학자 제임스 그레고리(James Gregory)와 18세기에서 콜린 매클로린(Colin Maclaurin)의 연구는 삼각 급수(trigonometric series)의 발전에 영향을 미쳤습니다.[27] 역시, 18세기에서, 브룩 테일러(Brook Taylor)는 일반적인 테일러 급수(Taylor series)를 정의했습니다.[28]
Trigonometric ratios

삼각 비율은 직각 삼각형의 변 사이의 비율입니다. 이들 비율은 알려진 각도 A의 다음 삼각 함수(trigonometric function)에 의해 제공되며, 여기서 a, b 및 c는 첨부된 그림에서 변의 길이를 참조합니다:
- 사인(Sine) 함수 (sin)는 빗변(hypotenuse)에 대한 각도의 대변의 비율로 정의됩니다.
- 코사인(Cosine) 함수 (cos)는 빗변에 대한 인접한(adjacent) 다리 (직각에 대한 각도를 인접하는 삼각형의 변)의 비율로 정의됩니다.
- 탄젠트(Tangent) 함수 (tan)는 인접한 다리에 대한 대각 다리의 비율로 정의됩니다.
빗변(hypotenuse)은 직각 삼각형에서 90도 각도의 반대 변입니다; 그것은 삼각형의 가장-긴 변이고 각도 A에 인접한 두 변 중 하나입니다. 인접한 다리(adjacent leg)는 각도 A에 인접한 나머지 다른 변입니다. 반대 변(opposite side)은 각도 A와 반대에 있는 변입니다. 용어 높이(perpendicular) 및 밑변(base)은 때때로, 각각, 반대 변과 인접한 면에 대해 사용됩니다. 니모닉(Mnemonics)에서 아래를 참조하십시오.
같은 예리한 각도 A를 갖는 임의의 두 직각 삼각형은 닮았으므로,[29] 삼각 비율의 값은 오직 각도 A에 의존합니다.
이들 함수의 역원(reciprocals)은, 각각, 코시컨트(cosecant, csc), 시컨트(secant, sec), 및 코탄젠트(cotangent, cot)로 이름 짓습니다:
코사인, 코탄젠트, 및 코시컨트는 그렇게 이름 지어졌는데 왜냐하면 그들은 "코-(co-)"로 축약된 보완 각도의 각각 사인, 탄젠트, 및 시컨트이기 때문입니다.[30]
이들 함수와 함께, 우리는 사인의 법칙(law of sines) 및 코사인의 법칙(law of cosines)을 사용함으로써 임의의 삼각형에 대한 거의 모든 질문에 대답할 수 있습니다.[31] 이들 법칙은 두 변과 그들의 포함된 각도 또는 두 각도와 한 변 또는 세 변이 알려진 즉시 임의의 삼각형의 남아있는 각도와 변을 계산하기 위해 사용될 수 있습니다.
Mnemonics
A common use of mnemonics is to remember facts and relationships in trigonometry. For example, the sine, cosine, and tangent ratios in a right triangle can be remembered by representing them and their corresponding sides as strings of letters. For instance, a mnemonic is SOH-CAH-TOA:[32]
- Sine = Opposite ÷ Hypotenuse
- Cosine = Adjacent ÷ Hypotenuse
- Tangent = Opposite ÷ Adjacent
One way to remember the letters is to sound them out phonetically (i.e., SOH-CAH-TOA, which is pronounced 'so-ka-toe-uh' /soʊkæˈtoʊə/). Another method is to expand the letters into a sentence, such as "Some Old Hippie Caught Another Hippie Trippin' On Acid".[33]
The unit circle and common trigonometric values

삼각 비율은 평면에서 원점에 중심을 둔 반지름 1의 원인 단위 원(unit circle)을 사용하여 역시 나타낼 수 있습니다. [34] 이 설정에서, 표준 위치(standard position)에서 위치된 각도 A의 종점 변(terminal side)은 및 인 점 (x,y)에서 단위 원과 교차할 것입니다.[34] 이 표현은 다음 테이블에서 그들처럼, 공통적으로 발견되는 삼각법 값의 계산에 대해 허용됩니다:[35]
| Function | 0 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| sine | 0 | 1 | 0 | ||||||
| cosine | 1 | 0 | -1 | ||||||
| tangent | 0 | undefined | 0 | ||||||
| secant | 1 | undefined | -1 | ||||||
| cosecant | undefined | 1 | undefined | ||||||
| cotangent | undefined | 0 | undefined |
Trigonometric functions of real or complex variables
단위 원(unit circle)을 사용하면, 우리는 삼각 비율의 정의를 모든 양 및 음의 인수로 확장할 수 있습니다[36] (삼각 함수(trigonometric function)를 참조하십시오).
Graphs of trigonometric functions
다음 테이블은 여섯 주요 삼각 함수의 그래프 속성을 요약한 것입니다:[37][38]
| 함수(Function) | 주기(Period) | 도메인(Domain) | 치역(Range) | 그래프(Graph) |
|---|---|---|---|---|
| sine | 
| |||
| cosine | 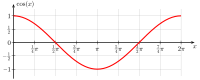
| |||
| tangent | 
| |||
| secant | 
| |||
| cosecant | 
| |||
| cotangent | 
|
Inverse trigonometric functions
여섯 주요 삼각 함수는 주기적이기 때문에, 그들은 단사적(injective) (또는, 1 대 1)이지 않고, 따라서 역-가능이 아닙니다. 삼각 함수의 도메인을 제한함(restricting)으로써, 어쨌든, 그들은 역-가능으로 만들 수 있습니다.[39]: 48ff
역 삼각 함수의 이름은, 그들의 도메인 및 치역과 함께, 다음 테이블에서 볼 수 있습니다:[39]: 48ff [40]: 521ff
| 이름(Name) | 보통 표기법(Usual notation) | 정의(Definition( | 실수 결과에 대해 x의 도메인 | 보통 주요 값의 치역 (라디안) |
보통 주요 값의 치역 (각도) |
|---|---|---|---|---|---|
| arcsine | y = arcsin(x) | x = sin(y) | −1 ≤ x ≤ 1 | −π/2 ≤ y ≤ π/2 | −90° ≤ y ≤ 90° |
| arccosine | y = arccos(x) | x = cos(y) | −1 ≤ x ≤ 1 | 0 ≤ y ≤ π | 0° ≤ y ≤ 180° |
| arctangent | y = arctan(x) | x = tan(y) | all real numbers | −π/2 < y < π/2 | −90° < y < 90° |
| arccotangent | y = arccot(x) | x = cot(y) | all real numbers | 0 < y < π | 0° < y < 180° |
| arcsecant | y = arcsec(x) | x = sec(y) | x ≤ −1 or 1 ≤ x | 0 ≤ y < π/2 or π/2 < y ≤ π | 0° ≤ y < 90° or 90° < y ≤ 180° |
| arccosecant | y = arccsc(x) | x = csc(y) | x ≤ −1 or 1 ≤ x | −π/2 ≤ y < 0 or 0 < y ≤ π/2 | −90° ≤ y < 0° or 0° < y ≤ 90° |
Power series representations
실수 변수의 함수로 여길 때, 삼각 비율은 무한 급수(infinite series)에 의해 표현될 수 있습니다. 예를 들어 사인과 코사인은 다음 표현을 가집니다:[41]
이들 정의와 함께, 삼각 함수는 복소수(complex number)에 대해 정의될 수 있습니다.[42] 실수 또는 복소수 변수의 함수로 확장될 때, 다음 공식(formula)은 복소 지수를 유지됩니다:
삼각 함수의 관점에서 쓰인, 이 복소 지수 함수는 특히 유용합니다.[43][44]
Calculating trigonometric functions
삼각 함수는 수학 테이블(mathematical table)에 대해 가장-초기 사용 중 하나였습니다.[45] 그러한 테이블은 수학 교과서에 통합되었고 학생들은 값을 찾는 것을 배웠고 더 높은 정확도를 얻기 위해 목록화된 값 사이의 보간(interpolate)하는 방법을 배웠습니다.[46] 미끄럼 자(Slide rule)는 삼각 함수에 대해 특수 스케일을 가집니다.[47]
공학용 계산기(Scientific calculator)는 주요 삼각 함수 (sin, cos, tan, 및 때때로 cis 및 그 역)을 계산하는 버튼이 있습니다.[48] 대부분의 것은 각도 측정 방법: 각도(degrees), 라디안, 및 때때로 그라디안(gradians)을 선택할 수 있습니다. 대부분의 컴퓨터 프로그래밍(programming language) 언어는 삼각 함수를 포함하는 함수 라이브러리를 제공합니다.[49] 대부분의 개인용 컴퓨터에 사용되는 마이크로 프로세서 칩에 통합된 부동 소수점 단위(floating point unit) 하드웨어는 삼각 함수 계산에 대한 내장 명령어를 가집니다.[50]
Other Trigonometric Functions
초기의 목록화된 여섯 비율 외에도, 비록 오늘날 거의 사용되지 않을지라도, 역사적으로 중요한 추가적인 삼각 함수가 있습니다. 이들은 현 (crd(θ) = 2 sin(θ/2)), 벌사인(versine) (versin(θ) = 1 − cos(θ) = 2 sin2(θ/2)) (이것은 가장-최초의 테이블에 나타났습니다[51]), 코벌사인(coversine) (coversin(θ) = 1 − sin(θ) = versin(π/2 − θ)), 헤벌사인(haversine) (haversin(θ) = 1/2versin(θ) = sin2(θ/2)),[52] 엑시컨트(exsecant) (exsec(θ) = sec(θ) − 1), 및 엑코시턴트(excosecant) (excsc(θ) = exsec(π/2 − θ) = csc(θ) − 1)를 포함합니다. 이들 함수 사이의 자세한 관계에 대해 삼각 항등식의 목록(List of trigonometric identities)을 참조하십시오.
Applications
Astronomy
수세기 동안, 구형 삼각법은 태양, 달, 항성 위치를 찾는 것,[53] 일식을 예측하는 것, 행성의 궤도를 묘사하는 것에 사용되어 왔습니다.[54]
현대에서, 삼각분할(triangulation)의 기술은 천문학(astronomy)에서 위성 항법 시스템(satellite navigation system)뿐만 아니라[16] 근처의 별까지 거리를 측정하기 위해 사용됩니다.[55]

역사적으로, 삼각법은 항해하는 선박의 위도와 경도를 찾고, 경로를 도면 위에 그리고, 및 항해 동안 거리를 계산하기 위해 사용되어 왔습니다.[56]
삼각법은 자율-주행 차량(autonomous vehicle)에 대해 인공 지능(artificial intelligence)과 전역 위치 시스템(Global Positioning System)에서 그러한 수단을 통해 항법에 여전히 사용됩니다.[57]
Surveying
토지 측량(surveying)에서, 삼각법은 물체 사이의 길이, 넓이 및 상대 각도를 계산에서 사용됩니다.[58]
더 큰 규모에서, 삼각법은 지리학(geography)에서 경계표 사이의 거리를 측정하기 위해 사용됩니다.[59]
Periodic functions

사인 및 코사인 함수는 음파 및 광파(light)를 설명하는 것과 같은 주기 함수(periodic function)의 이론에 기본입니다.[60] 푸리에(Fourier)는 모든 각 연속(continuous), 주기 함수(periodic function)가 삼각 함수의 무한 합(infinite sum)으로 설명될 수 있음을 발견했습니다.
심지어 비-주기 함수도 푸리에 변환(Fourier transform)을 통해 사인과 코사인의 적분(integral)으로 표현될 수 있습니다. 이것은 다른 분야들 사이에서 양자-역학[61] 및 통신[62]에 응용을 가집니다.
Optics and Acoustics
삼각법은 음향학(acoustics)[63] 및 광학(optics)[63]을 포함하여 많은 물리적 과학(physical science)에 유용합니다.[64] 이들 영역에서, 그들은 음파 및 광파를 설명하고, 경계- 및 전송-관련된 문제를 해결하기 위해 사용됩니다.[65]
Other applications
삼각법 또는 삼각 함수를 사용하는 다른 분야는 음악 이론(music theory),[66] 측지학(geodesy), 오디오 합성(audio synthesis),[67] 아키텍처(architecture),[68] 전자-공학(electronics),[66] 생물학(biology),[69] 의료 영상(medical imaging) ([CT scan|CT 스캔]] 및 초음파(ultrasound)),[70] 화학(chemistry),[71] 숫자 이론(number theory) (및 따라서 암호학(cryptology)),[72] 지진학(seismology),[63] 기상학(meteorology),[73] 해양학(oceanography),[74] 이미지 압축(image compression),[75] 음성학(phonetics),[76] 경제학(economics),[77] 전기 공학(electrical engineering), 기계 공학(mechanical engineering), 토목 공학(civil engineering),[66] 컴퓨터 그래픽(computer graphics),[78] 지도학(cartography),[66] 결정학(crystallography)[79] 및 게임 개발(game development)을 포함합니다.[78]
Identities

삼각법은 그의 많은 항등식, 즉, 모든 가능한 입력에 대해 참인 방정식에 대해 주목받아 왔습니다.[80]
오직 각도를 포함하는 항등식은 삼각 항등식으로 알려져 있습니다. 삼각형 항등식으로 알려진,[81] 다른 방정식은 주어진 삼각형의 변과 각도 모두를 관련시킵니다.
Triangle identities
다음 항등식에서, A, B 및 C는 삼각형의 각도이고 a, b 및 c는 (그림에서 보인 것처럼) 각각의 각도와 반대편의 삼각형의 변의 길이입니다.[82]
Law of sines
임의의 삼각형에 대해 ("sin 법칙"으로 역시 알려진) 사인의 법칙(law of sines)은 다음을 말합니다:[83]
여기서 는 삼각형의 넓이이고 R은 삼각형의 둘레-접하는 원(circumscribed circle)의 반지름입니다.
Law of cosines
(코사인 공식, 또는 "cos 법칙"으로 알려진) 코사인의 법칙(law of cosines)은 임의의 삼각형에 대한 피타고라스 정리의 확장입니다:[83]
또는 동등하게:
Law of tangents
프랑수아 비에트(François Viète)에 의해 개발된, 탄젠트의 법칙(law of tangents)은 삼각형의 미지수 변에 대해 풀 때 코사인의 법칙에 대한 대안이며, 삼각 테이블을 사용할 때 더 간단한 계산을 제공합니다.[84] 그것은 다음에 의해 제공됩니다:
Area
두 변 a와 b와 변 C 사이의 각도가 주어지면, 삼각형의 넓이는 두 변의 길이와 두 변 사이의 각도의 사인의 곱의 절반으로 제공됩니다:[83]
헤론의 공식(Heron's formula)은 삼각형의 넓이를 계산하기 위해 사용될 수 있는 또 다른 방법입니다. 이 공식은 만약 삼각형이 길이 a, b, 및 c의 변을 가지고, 만약 반-둘레가 다음이면,
삼각형의 넓이는 다음임을 말합니다:[85]
- ,
여기서 R은 삼각형의 둘레-원(circumcircle)의 반지름입니다.
Trigonometric identities
Pythagorean identities
다음 삼각 항등식(identities)은 피타고라스 정리(Pythagorean theorem)와 관련되고 임의의 값에 대해 유지됩니다:[86]
Euler's formula
임을 말하는, 오일러의 공식(Euler's formula)은 e와 허수 단위(imaginary unit) i에 관한 사인, 코사인, 및 탄젠트에 대해 다음의 해석적(analytical) 항등식을 생산합니다.
Other trigonometric identities
다른 공통적으로 사용되는 삼각 항등식은 반-각 항등식, 각도 합과 차이 항등식, 및 곱-에서-합 항등식을 포함합니다.[29]
See also
References
- ^ "trigonometry". Online Etymology Dictionary.
- ^ R. Nagel (ed.), Encyclopedia of Science, 2nd Ed., The Gale Group (2002)
- ^ Boyer, Carl Benjamin (1991). A History of Mathematics (2nd ed.). John Wiley & Sons, Inc. ISBN 978-0-471-54397-8.
- ^ Charles William Hackley (1853). A treatise on trigonometry, plane and spherical: with its application to navigation and surveying, nautical and practical astronomy and geodesy, with logarithmic, trigonometrical, and nautical tables. G. P. Putnam.
- ^ Mary Jane Sterling (24 February 2014). Trigonometry For Dummies. John Wiley & Sons. p. 185. ISBN 978-1-118-82741-3.
- ^ P.R. Halmos (1 December 2013). I Want to be a Mathematician: An Automathography. Springer Science & Business Media. ISBN 978-1-4612-1084-9.
- ^ Ron Larson; Robert P. Hostetler (10 March 2006). Trigonometry. Cengage Learning. p. 230. ISBN 0-618-64332-X.
- ^ Boyer (1991), p. 162, "Greek Trigonometry and Mensuration". sfnp error: multiple targets (2×): CITEREFBoyer1991 (help)
- ^ Aaboe, Asger (2001). Episodes from the Early History of Astronomy. New York: Springer. ISBN 0-387-95136-9
- ^ Otto Neugebauer (1975). A history of ancient mathematical astronomy. 1. Springer-Verlag. p. 744. ISBN 978-3-540-06995-9.
- ^ Thurston, pp. 235–236.
- ^ Toomer, G. (1998), Ptolemy's Almagest, Princeton University Press, ISBN 978-0-691-00260-6
- ^ Thurston, pp. 239–243.
- ^ Boyer p. 215
- ^ Gingerich, Owen. "Islamic astronomy." Scientific American 254.4 (1986): 74-83
- ^ a b Michael Willers (13 February 2018). Armchair Algebra: Everything You Need to Know From Integers To Equations. Book Sales. p. 37. ISBN 978-0-7858-3595-0.
- ^ "Al-Tusi_Nasir biography". MacTutor History of Mathematics archive. Retrieved 2018-08-05.
One of al-Tusi's most important mathematical contributions was the creation of trigonometry as a mathematical discipline in its own right rather than as just a tool for astronomical applications. In Treatise on the quadrilateral al-Tusi gave the first extant exposition of the whole system of plane and spherical trigonometry. This work is really the first in history on trigonometry as an independent branch of pure mathematics and the first in which all six cases for a right-angled spherical triangle are set forth.
- ^ "the cambridge history of science". October 2013.
- ^ electricpulp.com. "ṬUSI, NAṢIR-AL-DIN i. Biography – Encyclopaedia Iranica". www.iranicaonline.org. Retrieved 2018-08-05.
His major contribution in mathematics (Nasr, 1996, pp. 208-214) is said to be in trigonometry, which for the first time was compiled by him as a new discipline in its own right. Spherical trigonometry also owes its development to his efforts, and this includes the concept of the six fundamental formulas for the solution of spherical right-angled triangles.
- ^ "trigonometry". Encyclopædia Britannica. Retrieved 2008-07-21.
- ^ Berggren, J. Lennart (2007). "Mathematics in Medieval Islam". The Mathematics of Egypt, Mesopotamia, China, India, and Islam: A Sourcebook. Princeton University Press. p. 518. ISBN 978-0-691-11485-9.
- ^ Boyer pp. 237, 274
- ^ "Regiomontanus biography". History.mcs.st-and.ac.uk. Retrieved 2017-03-08.
- ^ N.G. Wilson (1992). From Byzantium to Italy. Greek Studies in the Italian Renaissance, London. ISBN 0-7156-2418-0
- ^ Grattan-Guinness, Ivor (1997). The Rainbow of Mathematics: A History of the Mathematical Sciences. W.W. Norton. ISBN 978-0-393-32030-5.
- ^ Robert E. Krebs (2004). Groundbreaking Scientific Experiments, Inventions, and Discoveries of the Middle Ages and the Renaissance. Greenwood Publishing Group. p. 153. ISBN 978-0-313-32433-8.
- ^ William Bragg Ewald (2007). From Kant to Hilbert: a source book in the foundations of mathematics. Oxford University Press US. p. 93. ISBN 0-19-850535-3
- ^ Kelly Dempski (2002). Focus on Curves and Surfaces. p. 29. ISBN 1-59200-007-X
- ^ a b James Stewart; Lothar Redlin; Saleem Watson (16 January 2015). Algebra and Trigonometry. Cengage Learning. p. 448. ISBN 978-1-305-53703-3.
- ^ Dick Jardine; Amy Shell-Gellasch (2011). Mathematical Time Capsules: Historical Modules for the Mathematics Classroom. MAA. p. 182. ISBN 978-0-88385-984-1.
- ^ Krystle Rose Forseth; Christopher Burger; Michelle Rose Gilman; Deborah J. Rumsey (7 April 2008). Pre-Calculus For Dummies. John Wiley & Sons. p. 218. ISBN 978-0-470-16984-1.
- ^ Weisstein, Eric W. "SOHCAHTOA". MathWorld.
- ^ A sentence more appropriate for high schools is "'Some Old Horse Came A''Hopping Through Our Alley". Foster, Jonathan K. (2008). Memory: A Very Short Introduction. Oxford. p. 128. ISBN 978-0-19-280675-8.
- ^ a b David Cohen; Lee B. Theodore; David Sklar (17 July 2009). Precalculus: A Problems-Oriented Approach, Enhanced Edition. Cengage Learning. ISBN 1-4390-4460-0.
- ^ W. Michael Kelley (2002). The Complete Idiot's Guide to Calculus. Alpha Books. p. 45. ISBN 978-0-02-864365-6.
- ^ Jenny Olive (18 September 2003). Maths: A Student's Survival Guide: A Self-Help Workbook for Science and Engineering Students. Cambridge University Press. p. 175. ISBN 978-0-521-01707-7.
- ^ Mary P Attenborough (30 June 2003). Mathematics for Electrical Engineering and Computing. Elsevier. p. 418. ISBN 978-0-08-047340-6.
- ^ Ron Larson; Bruce H. Edwards (10 November 2008). Calculus of a Single Variable. Cengage Learning. p. 21. ISBN 0-547-20998-3.
- ^ a b Elizabeth G. Bremigan; Ralph J. Bremigan; John D. Lorch (2011). Mathematics for Secondary School Teachers. MAA. ISBN 978-0-88385-773-1.
- ^ Martin Brokate; Pammy Manchanda; Abul Hasan Siddiqi (3 August 2019). Calculus for Scientists and Engineers. Springer. ISBN 9789811384646.
- ^ Serge Lang (14 March 2013). Complex Analysis. Springer. p. 63. ISBN 978-3-642-59273-7.
- ^ Silvia Maria Alessio (9 December 2015). Digital Signal Processing and Spectral Analysis for Scientists: Concepts and Applications. Springer. p. 339. ISBN 978-3-319-25468-5.
- ^ K. RAJA RAJESWARI; B. VISVESVARA RAO (24 March 2014). SIGNALS AND SYSTEMS. PHI Learning. p. 263. ISBN 978-81-203-4941-4.
- ^ John Stillwell (23 July 2010). Mathematics and Its History. Springer Science & Business Media. p. 313. ISBN 978-1-4419-6053-5.
- ^ Martin Campbell-Kelly; Professor Emeritus of Computer Science Martin Campbell-Kelly; Visiting Fellow Department of Computer Science Mary Croarken; Raymond Flood; Eleanor Robson (2 October 2003). The History of Mathematical Tables: From Sumer to Spreadsheets. OUP Oxford. ISBN 978-0-19-850841-0.
- ^ George S. Donovan; Beverly Beyreuther Gimmestad (1980). Trigonometry with calculators. Prindle, Weber & Schmidt. ISBN 978-0-87150-284-1.
- ^ Ross Raymond Middlemiss (1945). Instructions for Post-trig and Mannheim-trig Slide Rules. Frederick Post Company.
- ^ Bonnier Corporation (April 1974). Popular Science. Bonnier Corporation. p. 125.
- ^ Steven S Skiena; Miguel A. Revilla (18 April 2006). Programming Challenges: The Programming Contest Training Manual. Springer Science & Business Media. p. 302. ISBN 978-0-387-22081-9.
- ^ Intel® 64 and IA-32 Architectures Software Developer's Manual Combined Volumes: 1, 2A, 2B, 2C, 3A, 3B and 3C (PDF). Intel. 2013.
- ^ Boyer (1991, pp. xxiii–xxiv) harvtxt error: multiple targets (2×): CITEREFBoyer1991 (help)
- ^ Nielsen (1966, pp. xxiii–xxiv)
- ^ Olinthus Gregory (1816). Elements of Plane and Spherical Trigonometry: With Their Applications to Heights and Distances Projections of the Sphere, Dialling, Astronomy, the Solution of Equations, and Geodesic Operations. Baldwin, Cradock, and Joy.
- ^ Neugebauer, Otto. "Mathematical methods in ancient astronomy." Bulletin of the American Mathematical Society 54.11 (1948): 1013-1041.
- ^ Michael Seeds; Dana Backman (5 January 2009). Astronomy: The Solar System and Beyond. Cengage Learning. p. 254. ISBN 0-495-56203-3.
- ^ John Sabine (1800). The Practical Mathematician, Containing Logarithms, Geometry, Trigonometry, Mensuration, Algebra, Navigation, Spherics and Natural Philosophy, Etc. p. 1.
- ^ Mordechai Ben-Ari; Francesco Mondada (25 October 2017). Elements of Robotics. Springer. p. 16. ISBN 978-3-319-62533-1.
- ^ George Roberts Perkins (1853). Plane Trigonometry and Its Application to Mensuration and Land Surveying: Accompanied with All the Necessary Logarithmic and Trigonometric Tables. D. Appleton & Company.
- ^ Charles W. J. Withers; Hayden Lorimer (14 December 2015). Geographers: Biobibliographical Studies. A&C Black. p. 6. ISBN 978-1-4411-0785-5.
- ^ H. G. ter Morsche; J. C. van den Berg; E. M. van de Vrie (7 August 2003). Fourier and Laplace Transforms. Cambridge University Press. p. 61. ISBN 978-0-521-53441-3.
- ^ Bernd Thaller (8 May 2007). Visual Quantum Mechanics: Selected Topics with Computer-Generated Animations of Quantum-Mechanical Phenomena. Springer Science & Business Media. p. 15. ISBN 978-0-387-22770-2.
- ^ M. Rahman (2011). Applications of Fourier Transforms to Generalized Functions. WIT Press. ISBN 978-1-84564-564-9.
- ^ a b c John J. Schiller; Marie A. Wurster (1988). College Algebra and Trigonometry: Basics Through Precalculus. Scott, Foresman. ISBN 978-0-673-18393-4.
- ^ Lawrence Bornstein; Basic Systems, Inc (1966). Trigonometry for the Physical Sciences. Appleton-Century-Crofts.
- ^ Dudley H. Towne (5 May 2014). Wave Phenomena. Dover Publications. ISBN 978-0-486-14515-0.
- ^ a b c d E. Richard Heineman; J. Dalton Tarwater (1 November 1992). Plane Trigonometry. McGraw-Hill. ISBN 978-0-07-028187-5.
- ^ Mark Kahrs; Karlheinz Brandenburg (18 April 2006). Applications of Digital Signal Processing to Audio and Acoustics. Springer Science & Business Media. p. 404. ISBN 978-0-306-47042-4.
- ^ Kim Williams; Michael J. Ostwald (9 February 2015). Architecture and Mathematics from Antiquity to the Future: Volume I: Antiquity to the 1500s. Birkhäuser. p. 260. ISBN 978-3-319-00137-1.
- ^ Dan Foulder (15 July 2019). Essential Skills for GCSE Biology. Hodder Education. p. 78. ISBN 978-1-5104-6003-4.
- ^ Luciano Beolchi; Michael H. Kuhn (1995). Medical Imaging: Analysis of Multimodality 2D/3D Images. IOS Press. p. 122. ISBN 978-90-5199-210-6.
- ^ Marcus Frederick Charles Ladd (2014). Symmetry of Crystals and Molecules. Oxford University Press. p. 13. ISBN 978-0-19-967088-8.
- ^ Gennady I. Arkhipov; Vladimir N. Chubarikov; Anatoly A. Karatsuba (22 August 2008). Trigonometric Sums in Number Theory and Analysis. Walter de Gruyter. ISBN 978-3-11-019798-3.
- ^ Study Guide for the Course in Meteorological Mathematics: Latest Revision, Feb. 1, 1943. 1943.
- ^ Mary Sears; Daniel Merriman; Woods Hole Oceanographic Institution (1980). Oceanography, the past. Springer-Verlag. ISBN 978-0-387-90497-9.
- ^ "JPEG Standard (JPEG ISO/IEC 10918-1 ITU-T Recommendation T.81)" (PDF). International Telecommunications Union. 1993. Retrieved 6 April 2019.
- ^ Kirsten Malmkjaer (4 December 2009). The Routledge Linguistics Encyclopedia. Routledge. p. 1. ISBN 978-1-134-10371-3.
- ^ Kamran Dadkhah (11 January 2011). Foundations of Mathematical and Computational Economics. Springer Science & Business Media. p. 46. ISBN 978-3-642-13748-8.
- ^ a b Christopher Griffith (12 November 2012). Real-World Flash Game Development: How to Follow Best Practices AND Keep Your Sanity. CRC Press. p. 153. ISBN 978-1-136-13702-0.
- ^ John Joseph Griffin (1841). A System of Crystallography, with Its Application to Mineralogy. R. Griffin. p. 119.
- ^ Dugopolski (July 2002). Trigonometry I/E Sup. Addison Wesley. ISBN 978-0-201-78666-8.
- ^ V&S EDITORIAL BOARD (6 January 2015). CONCISE DICTIONARY OF MATHEMATICS. V&S Publishers. p. 288. ISBN 978-93-5057-414-0.
- ^ Lecture 3 | Quantum Entanglements, Part 1 (Stanford), Leonard Susskind, trigonometry in five minutes, law of sin, cos, euler formula 2006-10-09.
- ^ a b c Cynthia Y. Young (19 January 2010). Precalculus. John Wiley & Sons. p. 435. ISBN 978-0-471-75684-2.
- ^ Ron Larson (29 January 2010). Trigonometry. Cengage Learning. p. 331. ISBN 1-4390-4907-6.
- ^ Richard N. Aufmann; Vernon C. Barker; Richard D. Nation (5 February 2007). College Trigonometry. Cengage Learning. p. 306. ISBN 0-618-82507-X.
- ^ Peterson, John C. (2004). Technical Mathematics with Calculus (illustrated ed.). Cengage Learning. p. 856. ISBN 978-0-7668-6189-3. Extract of page 856
Bibliography
- Boyer, Carl B. (1991). A History of Mathematics (Second ed.). John Wiley & Sons, Inc. ISBN 978-0-471-54397-8.
- "Trigonometric functions", Encyclopedia of Mathematics, EMS Press, 2001 [1994]
- Christopher M. Linton (2004). From Eudoxus to Einstein: A History of Mathematical Astronomy. Cambridge University Press.
- Nielsen, Kaj L. (1966), Logarithmic and Trigonometric Tables to Five Places (2nd ed.), New York, USA: Barnes & Noble, LCCN 61-9103
- Weisstein, Eric W. "Trigonometric Addition Formulas". MathWorld.
External links
- Khan Academy: Trigonometry, free online micro lectures
- Trigonometry by Alfred Monroe Kenyon and Louis Ingold, The Macmillan Company, 1914. In images, full text presented.
- Benjamin Banneker's Trigonometry Puzzle at Convergence
- Dave's Short Course in Trigonometry by David Joyce of Clark University
- Trigonometry, by Michael Corral, Covers elementary trigonometry, Distributed under GNU Free Documentation License





































![{\displaystyle [-1,1]}](https://dawoum.duckdns.org/api/rest_v1/media/math/render/svg/51e3b7f14a6f70e614728c583409a0b9a8b9de01)

![{\displaystyle (-\infty ,-1]\cup [1,\infty )}](https://dawoum.duckdns.org/api/rest_v1/media/math/render/svg/7e3448e2de68557e598967fb8b1f8900260c4a64)











![{\displaystyle {\frac {a-b}{a+b}}={\frac {\tan \left[{\tfrac {1}{2}}(A-B)\right]}{\tan \left[{\tfrac {1}{2}}(A+B)\right]}}}](https://dawoum.duckdns.org/api/rest_v1/media/math/render/svg/a1da4e06eb6f25cd7f7fc1a7784a11a82ae53f9f)







