Locus (mathematics)

기하학(geometry)에서, 자취(locus, 복수형: loci, "장소", "위치"에 대한 라틴어 단어)는 그것의 위치가 하나 이상의 지정된 조건에 의해 만족시키거나 결정되는 모든 점 (공통적으로, 직선, 선분, 곡선, 또는 표면)의 집합(set)입니다.^[1]^[2]

다시 말해서, 어떤 속성을 만족시키는 점들의 집합은 종종 이 성질을 만족시키는 점의 자취(locus of a point)라고 불립니다. 이 공식에서 단수를 사용한 것은 19세기 말까지 수학자들이 무한 집합을 고려하지 않았다는 증거입니다. 그들은 직선과 곡선을 점의 집합으로 보는 대신 점이 위치하거나 이동할 수 있는 장소로 보았습니다.

History and philosophy

20세기 초까지, 기하학적 모양 (예를 들어, 곡선)은 점의 무한한 집합으로 고려되지 않았습니다; 오히려, 그것은 점이 위치되거나 점이 이동하는 엔터디로 생각되었습니다. 따라서 유클리드 평면(Euclidean plane)에서 원은 원의 고정된 점, 원의 중심에서 주어진 거리에 있는 점의 자취로 정의되었습니다. 현대 수학에서, 유사한 개념은 모양을 집합으로 설명함으로써 더 자주 재구성될니다; 예를 들어, 원이 중심에서 주어진 거리에 있는 점들의 집합이라고 말합니다.^[3]

집합-이론적 관점과 대조적으로, 오래된 형식화는 실제 무한을 피하는 것이 초기 수학자들의 중요한 철학적 입장이었기 때문에 무한 모음을 고려하는 것을 피합니다.^[4]^[5]

일단 집합 이론이 전체 수학이 만들어지는 보편적인 기초가 되자,^[6] 자취라는 용어는 다소 구식으로 바뀌었습니다.^[7] 그럼에도 불구하고, 그 단어는 주로 간결한 형식화를 위해 여전히 널리 사용됩니다. 예를 들어:

임계 자취(Critical locus), 미분-가능 함수(differentiable function)의 임계점(critical points)의 집합.
영 자취 또는 사라지는 자취, 값(value) 영을 취한다는 점에서 함수가 사라지는 점의 집합.
특이 자취(Singular locus), 대수적 다양체(algebraic variety)의 특이점(singular points)의 집합.
연결성 자취(Connectedness locus), 함수의 줄리아 집합(Julia set)이 연결된 유리 함수(rational functions)의 가족의 매개변수 집합의 부분집합.

보다 최근에는, 스킴(schemes)의 이론과 같은 기술과 수학의 기초를 제공하기 위해 집합 이론(set theory) 대신 카테고리 이론(category theory)의 사용은 점의 집합이라기보다는 그 자체로 대상으로서의 자취의 원래 정의와 더 유사한 개념으로 돌아왔습니다.^[5]

Examples in plane geometry

평면 기하학에서 예제는 다음을 포함합니다:

두 점에서 등거리에 있는 점의 집합은 두 점을 연결하는 선분(line segment)에 대한 수직 이등분선(perpendicular bisector)입니다.^[8]
교차하는 두 직선에서 등거리에 있는 점의 집합은 각도 이등분선(angle bisector)입니다.
모든 원뿔 단면(conic sections)은 자취입니다:^[9]
- 원(Circle): 단일 점으로부터의 거리가 상수 (반지름)인 점의 집합.
- 포물선(Parabola): 고정된 점 (초점)과 직선 (방향선)에서 등거리에 있는 점의 집합.
- 쌍곡선(Hyperbola): 두 개의 주어진 초점까지의 거리 사이 차이의 절댓값이 상수인 각각에 대한 점의 집합.
- 타원(Ellipse): 두 개의 주어진 초점까지의 거리의 합이 상수인 각 점의 집합.

자취의 다른 예제는 수학의 다양한 영역에서 나타납니다. 예를 들어, 복소 동역학(complex dynamics)에서, 망델브로 집합(Mandelbrot set)은 다항식 맵의 가족의 연결성 자취(connectedness locus)로 특성화될 수 있는 복소 평면(complex plane)의 부분집합입니다.

Proof of a locus

기하학적 모양이 주어진 조건의 집합에 대한 올바른 자취임을 입증하기 위해, 일반적으로 증명을 두 단계로 나눕니다:^[10]

조건을 만족시키는 모든 점이 주어진 모양 위에 있음을 증명.
주어진 모양 위의 모든 점이 조건을 만족시킨다는 증명.

Examples

First example

두 개의 주어진 점에 대한 거리 k = d₁/d₂의 주어진 비율을 가지는 점 P의 자취를 찾으십시오.

이 예제에서 k = 3, A(−1, 0), 및 B(0, 2)가 고정된 점으로 선택됩니다.

P(x, y)는 자취의 점입니다

\Leftrightarrow |PA|=3|PB|

\Leftrightarrow |PA|^{2}=9|PB|^{2}

\Leftrightarrow (x+1)^{2}+(y-0)^{2}=9(x-0)^{2}+9(y-2)^{2}

\Leftrightarrow 8(x^{2}+y^{2})-2x-36y+35=0

\Leftrightarrow \left(x-{\frac {1}{8}}\right)^{2}+\left(y-{\frac {9}{4}}\right)^{2}={\frac {45}{64}}.

이 방정식은 중심 (1/8, 9/4)과 반지름 ${\tfrac {3}{8}}{\sqrt {5}}$ 를 갖는 원(circle)을 나타냅니다. 그것은 k, A, 및 B의 이들 값에 의해 정의된 아폴로니우스의 원(circle of Apollonius)입니다.

Second example

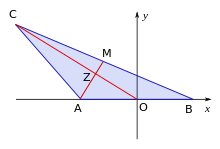
삼각형 ABC는 길이 c를 갖는 고정된 변 [AB]를 가지고 있습니다. A와 C의 중앙선(medians)이 직교(orthogonal)임을 만족하는 세 번째 꼭짓점(vertex) C의 자취를 결정합니다.

A(−c/2, 0), B(c/2, 0)를 만족하는 직교정규(orthonormal) 좌표 시스템(coordinate system)을 선택합니다. C(x, y)는 변수 세 번째 꼭짓점입니다. [BC]의 중심은 M((2x + c)/4, y/2)입니다. C로부터 중앙선은 기울기 y/x를 가집니다. 중앙선 AM은 기울기(slope) 2y/(2x + 3c)를 가집니다.

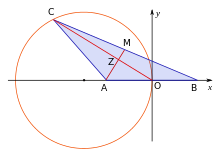
C(x, y)는 자취의 점입니다

\Leftrightarrow

A와 C에서 중앙선은 직교합니다

\Leftrightarrow {\frac {y}{x}}\cdot {\frac {2y}{2x+3c}}=-1

\Leftrightarrow 2y^{2}+2x^{2}+3cx=0

\Leftrightarrow x^{2}+y^{2}+(3c/2)x=0

\Leftrightarrow (x+3c/4)^{2}+y^{2}=9c^{2}/16.

꼭짓점 C의 자취는 중심 (−3c/4, 0)과 반지름 3c/4을 갖는 원입니다.

Third example

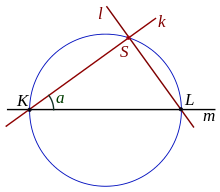
자취는 하나의 공통 매개변수(parameter)에 의존하는 두 개의 결합된 곡선에 의해 정의될 수도 있습니다. 만약 매개변수가 변하면, 결합된 곡선의 교차점이 자취를 설명합니다.

그림에서, 점 K와 L은 주어진 직선 m 위에 고정된 점입니다. 직선 k는 K를 통과하는 변수 직선입니다. L을 통과하는 직선 l은 k에 수직(perpendicular)입니다. k와 m 사이의 각도 $\alpha$ 는 매개변수입니다. k와 l은 공통 매개변수에 의존하는 결합된 직선입니다. k와 l의 변수 교차점 S는 원을 나타냅니다. 이 원은 두 개의 결합된 직선의 교차점의 자취입니다.

Fourth example

점의 자취는 일-차원일 필요가 없습니다 (원, 직선 등). 예를 들어,^[1] 부등식 $2 x + 3 y - 6 < 0$ 의 자취는 방정식 $2 x + 3 y - 6 = 0$ 의 직선 아래에 있는 평면 부분입니다.

References

^ ^a ^b James, Robert Clarke; James, Glenn (1992), Mathematics Dictionary, Springer, p. 255, ISBN 978-0-412-99041-0.
^ Whitehead, Alfred North (1911), An Introduction to Mathematics, H. Holt, p. 121, ISBN 978-1-103-19784-2.
^ Cooke, Roger L. (2012), "38.3 Topology", The History of Mathematics: A Brief Course (3rd ed.), John Wiley & Sons, ISBN 9781118460290, The word locus is one that we still use today to denote the path followed by a point moving subject to stated constraints, although, since the introduction of set theory, a locus is more often thought of statically as the set of points satisfying a given collection.
^ Bourbaki, N. (2013), Elements of the History of Mathematics, translated by J. Meldrum, Springer, p. 26, ISBN 9783642616938, the classical mathematicians carefully avoided introducing into their reasoning the 'actual infinity'.
^ ^a ^b Borovik, Alexandre (2010), "6.2.4 Can one live without actual infinity?", Mathematics Under the Microscope: Notes on Cognitive Aspects of Mathematical Practice, American Mathematical Society, p. 124, ISBN 9780821847619.
^ Mayberry, John P. (2000), The Foundations of Mathematics in the Theory of Sets, Encyclopedia of Mathematics and its Applications, vol. 82, Cambridge University Press, p. 7, ISBN 9780521770347, set theory provides the foundations for all mathematics.
^ Ledermann, Walter; Vajda, S. (1985), Combinatorics and Geometry, Part 1, Handbook of Applicable Mathematics, vol. 5, Wiley, p. 32, ISBN 9780471900238, We begin by explaining a slightly old-fashioned term.
^ George E. Martin, The Foundations of Geometry and the Non-Euclidean Plane, Springer-Verlag, 1975.
^ Hamilton, Henry Parr (1834), An Analytical System of Conic Sections: Designed for the Use of Students, Springer.
^ G. P. West, The new geometry: form 1.

[James-1] James, Robert Clarke; James, Glenn (1992), Mathematics Dictionary, Springer, p. 255, ISBN 978-0-412-99041-0.

[2] Whitehead, Alfred North (1911), An Introduction to Mathematics, H. Holt, p. 121, ISBN 978-1-103-19784-2.

[3] Cooke, Roger L. (2012), "38.3 Topology", The History of Mathematics: A Brief Course (3rd ed.), John Wiley & Sons, ISBN 9781118460290, The word locus is one that we still use today to denote the path followed by a point moving subject to stated constraints, although, since the introduction of set theory, a locus is more often thought of statically as the set of points satisfying a given collection.

[4] Bourbaki, N. (2013), Elements of the History of Mathematics, translated by J. Meldrum, Springer, p. 26, ISBN 9783642616938, the classical mathematicians carefully avoided introducing into their reasoning the 'actual infinity'.

[microscope-5] Borovik, Alexandre (2010), "6.2.4 Can one live without actual infinity?", Mathematics Under the Microscope: Notes on Cognitive Aspects of Mathematical Practice, American Mathematical Society, p. 124, ISBN 9780821847619.

[6] Mayberry, John P. (2000), The Foundations of Mathematics in the Theory of Sets, Encyclopedia of Mathematics and its Applications, vol. 82, Cambridge University Press, p. 7, ISBN 9780521770347, set theory provides the foundations for all mathematics.

[7] Ledermann, Walter; Vajda, S. (1985), Combinatorics and Geometry, Part 1, Handbook of Applicable Mathematics, vol. 5, Wiley, p. 32, ISBN 9780471900238, We begin by explaining a slightly old-fashioned term.

[8] George E. Martin, The Foundations of Geometry and the Non-Euclidean Plane, Springer-Verlag, 1975.

[9] Hamilton, Henry Parr (1834), An Analytical System of Conic Sections: Designed for the Use of Students, Springer.

[10] G. P. West, The new geometry: form 1.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]