Jump to content

Geometry

This is a fully translated article. Click here for more information.
From DawoumWiki, the free Mathematics self-learning
Original article: w:Geometry
For other uses, see Geometry (disambiguation).
Geometry
Projecting a sphere to a plane
Branches
  • Concepts
  • Features
Dimension
Zero-dimensional
One-dimensional
Two-dimensional
Triangle
Parallelogram
Quadrilateral
Circle
Three-dimensional
Four- / other-dimensional
Geometers
by name
by period
BCE
1–1400s
1400s–1700s
1700s–1900s
Present day
An illustration of Desargues' theorem, a result in Euclidean and projective geometry

기하학 (고대 그리스어 γεωμετρία (geōmetria) '토지 측정', γῆ (gê) '지구, 토지', 및 μέτρον (métron) '측정'에서 유래)은, 산술(arithmetic)과 함께, 수학(mathematics)의 가장 오래된 분야 중 하나입니다. 그것은 도형의 거리, 모양, 크기, 및 상대적인 위치와 관련된 공간의 속성에 관한 것입니다.[1] 기하학의 분야에서 일하는 수학자들은 기하학자(geometer)라고 불립니다.

19세기까지, 기하학은 점(point), 직선(line), 평면(plane), 거리(distance), 각도(angle), 표면(surface), 및 곡선(curve)의 개념을 기본 개념으로 포함하는 유클리드 기하학(Euclidean geometry)에[a] 거의 독점적으로 전념했습니다.[2]

19세기 동안, 여러 발견으로 기하학의 범위가 극적으로 확대되었습니다. 가장 오래된 그러한 발견 중 하나는 표면의 가우스 곡률(Gaussian curvature)이 유클리드 공간(Euclidean space)에서 임의의 특정 삽입(embedding)과 무관하다고 대략적으로 주장하는 가우스(Gauss)의 테오레마 에그레기움(Theorema Egregium) ("놀랄만한 정리")입니다. 이것은 표면이 본질적으로, 즉 독립된 공간으로 연구될 수 있음을 의미하고, 매니폴드(manifold)의 이론과 리만 기하학(Riemannian geometry)으로 확장되어 왔습니다.

19세기 후반에, 그것은 평행 공준(parallel postulate)없이 기하학 (비-유클리드 기하학(non-Euclidean geometries))은 임의의 모순을 도입없이 개발될 수 있는 것으로 나타났습니다. 일반 상대성(general relativity)의 기초가 되는 기하학은 비-유클리드 기하학의 유명한 응용입니다.

그 이후로, 기하학의 범위는 크게 확장되었고, 그 분야는 놓여있는 방법—미분 기하학(differential geometry), 대수적 기하학(algebraic geometry), 계산적 기하학(computational geometry), 대수적 토폴로지(algebraic topology), 이산 기하학(discrete geometry) (역시 조합론적 기가학으로 알려져 있음), 등.— 또는 무시되는 유클리드 공간의 속성–오직 점의 정렬을 고려하고 거리와 평행성은 고려하지 않는 투영 기하학(projective geometry), 각도와 거리의 개념을 생략하는 아핀 기하학(affine geometry), 연속성(continuity)을 생략하는 유한 기하학(finite geometry), 등에 의존하는 많은 하위분야로 분리되어 왔습니다.

원래 물리적 세계를 모델링하기 위해 개발된, 기하학은 거의 모든 과학(science)과 역시 예술(art), 건축(architecture), 및 그래픽(graphics)과 관련된 기타 활동에 응용을 가집니다.[3] 기하학은 역시 명백히 관련이 없는 수학의 분야에서 응용을 가집니다. 예를 들어, 대수적 기하학의 방법은 기본 산술(elementary arithmetic)의 관점에서 언급된 문제이고, 몇 세기 동안 미해결 상태로 남겨졌던 페라마의 마지막 정리(Fermat's Last Theorem)의 와일스의 증명(Wiles's proof)에서 기본적입니다.

History

Main article: History of geometry
A European and an Arab practicing geometry in the 15th century

기하학의 가장 초기에 기록된 시작은 기원전 2천년에서 고대 메소포타미아(Mesopotamia)와 이집트(Egypt)로 추적될 수 있습니다.[4][5] 초기 기하학은 측량(surveying), 건설(construction), 천문학(astronomy), 및 다양한 공예품에서 일부 실제 요구를 충족시키기 위해 개발되었던 길이, 각도, 넓이, 및 부피에 관련하는 경험적으로 발견된 원리의 모음이었습니다. 기하학에 대한 가장 초기의 알려진 텍스트는 이집트(Egyptian)의 린드 파피루스(Rhind Papyrus) (기원전 2000–1800년)와 모스코우 파피루스(Moscow Papyrus) (기원전 1890년경), 및 플림프턴 322(Plimpton 322) (기원전 1900)와 같은 바빌론의 점토 태블릿입니다. 예를 들어, 모스코우 파피루스는 잘린 피라미드, 또는 절두체(frustum)의 부피를 계산하는 공식을 제공합니다.[6] 후기의 점토 태블릿 (기원전 350–50년)은 바빌론의 천문학자들이 시간-속도 공간 내에서 목성의 위치와 운동(motion)을 계산하기 위해 사다리꼴(trapezoid) 절차를 구현했음을 보여줍니다. 이들 기하학적 절차는 평균 속력 정리(mean speed theorem)를 포함하여 옥스포드 계산기를 14세기까지 앞서 있었습니다.[7] 이집트 남부의 고대 누비아인(ancient Nubians)은 초기 버전의 해시계를 포함한 기하학의 시스템을 확립했습니다.[8][9]

기원전 7세기에, 그리스(Greek) 수학자 밀레토스의 탈레스(Thales of Miletus)는 피라미드의 높이와 해안에서 선박의 거리를 계산하는 것과 같은 문제를 해결하기 위해 기하학을 사용했습니다. 그는 탈레스의 정리(Thales' theorem)에 넷의 따름정리를 유도함으로써 기하학에 적용된 연역적 추론의 첫 번째 사용으로 공인되었습니다.[10] 피타고라스는 피타고라스 정리(Pythagorean theorem)의 첫 번째 증명으로 인정되었던 피타고라스 학파(Pythagorean School)를 설립했지만,[11] 그 정리의 명제는 오랜 역사를 가지고 있습니다.[12][13] 에우독수스(Eudoxus) (기원전 408–c. 355)는 소진의 방법(method of exhaustion)을 개발했으며, 이것은 곡선 도형의 넓이와 부피를 계산할 수 있을 뿐만 아니라,[14] 비-정수비율가능 크기(incommensurable magnitudes)의 문제를 피하는 비율의 이론을 개발하여, 후속 기하학자들에게 중요한 진전을 가능하게 했습니다. 기원전 300년경에, 기하학은 유클리드에 의해 혁명적으로 발전하며, 그의 원론(Elements)은 공리적 방법(axiomatic method)을 통해 수학적 엄격함(mathematical rigor)을 도입했었고 오늘날 수학에서 여전히 사용되는 형식의 가장 초기 예제, 정의, 공리, 정리, 및 증명의 예제로, 역사상 가장 성공적이고 영향력 있는 교과서로 널리 인정받습니다. 비록 원론의 내용의 대부분은 이미 알려져 있었지만, 유클리드는 그것을 하나의 일관된 논리적 프레임워크로 정리했습니다.[15] 원론은 20세기 중반까지 서구에서 모든 교육받은 사람들에게 알려져 있었고 그것의 내용은 여전히 오늘날에도 기하학 수업에서 가르쳐지고 있습니다.[16] 시러큐스(Syracuse)의 아르키메데스(Archimedes) (기원전 c. 287–212)는 소진의 방법(method of exhaustion)을 무한 급수의 합으로 포물선(parabola)의 호 아래 넓이(area)를 계산하기 위해 사용했고 파이(pi)의 놀라울 정도로 정확한 근사치를 제공했습니다.[17] 그는 역시 자신의 이름을 딴 나선(spiral)을 연구했고 회전의 표면(surfaces of revolution)의 부피(volume)에 대해 공식을 얻었습니다.

Woman teaching geometry. Illustration at the beginning of a medieval translation of Euclid's Elements, (c. 1310).

인도(Indian) 수학자들은 역시 기하학에 많은 중요한 공헌을 했습니다. 사타파타 브라마나(Satapatha Brahmana) (기원전 3세기)는 술바 수트라스(Sulba Sutras)와 유사한 의식으로서의 기하학적 구조에 대해 규칙이 포함되어 있습니다.[18] (Hayashi 2005, p. 363)에 따르면, Śulba Sūtras는 "비록 피타고라스 정리가 고대 바빌로니아인들에게 이미 알려졌음에도 불구하고, 세계에서 그것의 가장 오래된 현존하는 구두 표현"을 포함하고 있습니다. 그것들은 피타고라스 세-쌍(Pythagorean triple)의 목록을 포함하고 있으며,[19] 이것은 디오판토스 방정식(Diophantine equations)의 특별한 경우입니다.[20] 바크샬리 원고(Bakhshali manuscript)에서, (불규칙한 고체의 부피에 대한 문제를 포함하는) 몇 개의 기하학적 문제가 있습니다. 바크샬리 원고는 역시 "영에 대해 점을 갖는 십진 자리 값 시스템을 사용합니다."[21] 아리아바타(Aryabhata)의 아리아바티아(Aryabhatiya) (499)는 넓이와 부피의 계산을 포함합니다. 브라마굽타(Brahmagupta)는 628년에 그의 천문학 연구 Brāhma Sphuṭa Siddhānta를 저술했습니다. 66개의 산스크리트(Sanskrit) 구절을 포함하는, 12장은 둘의 섹션: "기본 연산" (세제곱근 포함, 분수, 비율과 비례, 및 물물교환을 포함)과 "실용 수학" (혼합, 수학 급수, 평면 도형, 벽돌 쌓기, 목재 톱질, 및 곡물 쌓기를 포함)으로 나뉘어 있습니다.[22] 후자의 섹션에서, 그는 순환 사변형(cyclic quadrilateral)의 대각선에 대한 그의 유명한 정리를 말했습니다. 12장은 역시 순환 사변형의 넓이에 대한 공식 (헤론의 공식(Heron's formula)의 일반화)과 마찬가지로 유리수 삼각형(rational triangle) (즉, 유리수 변과 유리수 넓이를 갖는 삼각형)에 대해 완전한 설명을 포함했습니다.[22]

중세(Middle Ages)에서, 중세 이슬람에서 수학(mathematics in medieval Islam)은 기하학, 특히 대수적 기하학(algebraic geometry)의 발전에 기여했습니다.[23][24] 알-마하니(Al-Mahani) (b. 853)는 정육면체를 대수학 문제로 복제하는 것과 같은 기하학적 문제를 줄이는 아이디어를 고안했습니다.[25] 타비트 이븐 커라(Thabit ibn Qurra) (라틴어로 Thebit로 알려짐) (836–901)는 기하학적 양의 비율(ratio)에 적용되는 산술(arithmetic) 연산을 다루었고, 해석적 기하학(analytic geometry)의 발전에 기여했습니다.[26] 오마르 카야얌(Omar Khayyám) (1048–1131)은 삼차 방정식(cubic equation)에 대한 기하학적 해를 찾았습니다.[27] 램버트 사변형(Lambert quadrilateral)과 사케리 사변형(Saccheri quadrilateral)을 포함하는 사변형(quadrilateral)에 대한 이븐 알-하이삼(Ibn al-Haytham) (알하젠), 오마르 카야얌과 나시르 알-딘 알-투시(Nasīr al-Dīn al-Tūsī)의 정리는 쌍곡선 기하학(hyperbolic geometry)에서 초기 결과였고, 플레이페어의 공리(Playfair's axiom)와 같은 대안적 공준과 함께, 이들 연구가 비텔롱(Witelo) (c. 1230-c. 1314), 게르소니데스(Gersonides) (1288-1344), 알폰소(Alfonso), [[John Wallis|존 월리스(John Wallis)], 및 조반니 지롤라모 사케리(Giovanni Girolamo Saccheri)를 포함한 후기 유럽 기하학자들 사이에서 비-유클리드 기하학의 발전에 상당한 영향을 미쳤습니다.[28]

17세기 초에, 기하학에서 두 가지 중요한 발전이 있었습니다. 첫 번째는 르네 데카르트(René Descartes) (1596–1650)와 피에르 드 페르마(Pierre de Fermat) (1601–1665)에 의한 해석적 기하학, 또는 좌표(coordinates)와 방정식(equation)을 갖는 기하학의 생성이었습니다.[29] 이것은 미적분학(calculus)의 발전과 물리학(physics)의 정확한 양적 과학에 필요한 전조 현상이었습니다.[30] 이 시기의 두 번째 기하학적 발전은 지라르 데자그르(Girard Desargues) (1591–1661)의 투영 기하학(projective geometry)의 시스템적인 연구였습니다.[31] 투영 기하학은 특히 그것들이 예술적 원근(artistic perspective)과 관련했기 때문에 투영(projections)과 단면(sections) 아래에서 변경되지 않는 모양의 속성을 연구합니다.[32]

19세기에 기하학에서 두 가지 발전은 그것이 이전에 연구되어 왔던 방법을 바꾸었습니다.[33] 이것들은 니콜라이 이바노비치 로바쳅스키(Nikolai Ivanovich Lobachevsky), 보여이 야노시(János Bolyai), 및 카를 프리드리히 가우스(Carl Friedrich Gauss)에 의한 비-유클리드 기하학(non-Euclidean geometries)의 발견과 펠릭스 클라인(Felix Klein)의 Erlangen Programme (이것은 유클리드 기하학과 비-유클리드 기하학을 일반화함)에서 중심 고려 사항으로서 대칭(symmetry)의 공식화였습니다. 당시의 두 명의 기하학의 대가는 주로 수학적 해석학(mathematical analysis)에서 도구로 연구하고, 리만 표면(Riemann surface)을 도입하는 베른하르트 리만(Bernhard Riemann) (1826–1866)과, 대수적 토폴로지(algebraic topology)와 동역학적 시스템(dynamical system)의 기하학 이론을 창시자 앙리 푸앵카레(Henri Poincaré)였습니다. 기하학의 개념에서 이들 주요 변화의 결과로써, "공간"의 개념은 풍부하고 다양한 것이 되었고, 복소 해석학(complex analysis)과 고전 역학(classical mechanics)만큼 다른 이론에 대해 자연스러운 배경이 되었습니다.[34]

Main concepts

다음은 기하학에서 가장 중요한 개념 중 일부입니다.[2][35][36]

Axioms

An illustration of Euclid's parallel postulate
See also: Euclidean geometry and Axiom

유클리드(Euclid)는 지금까지 쓰인 가장 영향력 있는 책 중 하나인 그의 원론(Elements)에서[37] 기하학에 대한 추상적인 접근을 취했습니다.[38] 유클리드는 점, 직선, 및 평면의 주요 또는 자명한 속성을 표현하는 특정 공리(axiom), 또는 공준(postulate)을 도입했습니다.[39] 그는 수학적 추론에 의해 다른 속성들을 엄격하게 연역하기 시작했습니다. 기하학에 대한 유클리드의 접근 방식의 특징은 엄격함이고, 공리적 기하학 또는 합성적(synthetic) 기하학으로 알려지게 되었습니다.[40] 19세기 초에서, 니콜라이 이바노비치 로바쳅스키(Nikolai Ivanovich Lobachevsky) (1792–1856), 보여이 야노시(János Bolyai) (1802–1860), 및 카를 프리드리히 가우스(Carl Friedrich Gauss) (1777–1855), 등에 의한 비-유클리드 기하학(non-Euclidean geometries)의 발견은 이 분야에 대한 관심의 부활로 이어졌고,[41] 20세기에 다비트 힐베르트(David Hilbert) (1862–1943)는 기하학의 현대적 기초를 제공하려는 시도에서 공리적 추론을 사용했습니다.[42]

Objects

Points

Main article: Point (geometry)

점은 일반적으로 건물 기하학에 대해 기본 대상으로 고려됩니다. 그것들은 유클리드의 정의에서 처럼 "부분을 가지지 않는 것",[43] 또는 합성 기하학(synthetic geometry)에서 처럼, 그것들이 반드시 가져야 하는 속성에 의해 정의될 수 있습니다. 현대 수학에서, 그것들은 일반적으로 자체로 공리적(axiomatically)으로 정의되는 공간(space)이라고 불리는 집합(set)의 원소(elements)로 정의됩니다.

이들 현대 정의와 함께, 모든 각 기하학적 모양은 점의 집합으로 정의됩니다; 이것은 한 직선이 통과하는 점의 집합으로 보이는 않는 또 다른 기본 객체인 합성 기하학에서 그 경우가 아닙니다.

어쨌든, 점이 원시 대상이 아니거나, 심지어 점없이 현대 기하학을 가집니다.[44][45] 가장 오래된 그러한 기하학 중 하나는 1919–1920년에 알프레드 노스 화이트헤드(Alfred North Whitehead)에 의해 공식화된 화이트헤드의 점-없는 기하학(Whitehead's point-free geometry)입니다.

Lines

Main article: Line (geometry)

유클리드(Euclid)는 직선을 "그 자체 위에 점에 관해 같게 놓여있는" "너비없는 길이"로 설명했습니다.[43] 현대 수학에서, 기하학의 다양성을 고려할 때, 직선의 개념은 기하학이 설명되는 방법과 밀접하게 연결되어 있습니다. 예를 들어, 해석적 기하학(analytic geometry)에서, 평면에서 한 직선은 종종 그것의 좌표가 주어진 선형 방정식(linear equation)을 만족시키는 점의 집합으로 정의되지만,[46] 입사 기하학(incidence geometry)과 같은 보다 추상적인 설정에서, 한 직선은 독립적인 대상일 수 있으며, 그것 위에 놓이는 점의 집합과 구별됩니다.[47] 미분 기하학에서, 측지선(geodesic)은 곡선화된 공간(curved spaces)에 대한 직선 개념의 일반화입니다.[48]

Planes

Main article: Plane (geometry)

유클리드 기하학에서, 평면(plane)은 무한하게 확장되는 평평한, 이-차원 표면입니다;[43] 다른 유형의 기하학에 대해 정의는 그것의 일반화입니다. 평면은 기하학의 많은 영역에서 사용됩니다. 예를 들어, 평면은 거리 또는 각도에 대한 참조없이 토폴로지적 표면(topological surface)으로 연구될 수 있습니다;[49] 그것은 공선성과 비율이 연구될 수 있지만 거리가 연구될 수 없는 아핀 공간(affine space)으로 연구될 수 있습니다;[50] 그것은 복소 해석학(complex analysis)의 기법을 사용하여 복소 평면(complex plane)으로 연구될 수 있습니다;[51] 기타 등등.

Angles

Main article: Angle

유클리드(Euclid)는 평면 각도(angle)를 평면에서 서로 만나고, 서로에 관해 직선에 놓이지 않은 두 직선의 서로에 대한 기울기로 정의합니다.[43] 현대 용어에서, 각도는 각도의 꼭짓점(vertex)이라고 불리는 공통 끝점을 공유하는 각도의 변이라고 불리는 둘의 반직선(rays)에 의해 형성된 그림입니다.[52]

Acute (a), obtuse (b), and straight (c) angles. The acute and obtuse angles are also known as oblique angles.

유클리드 기하학(Euclidean geometry)에서, 각도는 다각형(polygon)과 삼각형(triangle)을 연구하고, 마찬가지로 그것들의 권리 안에서 연구 대상을 형성하기 위해 사용됩니다.[43] 삼각형의 각도 또는 단위 원(unit circle)의 각도의 연구는 삼각법(trigonometry)의 기초를 형성합니다.[53]

미분 기하학(differential geometry)과 미적분학(calculus)에서, 평면 곡선(plane curve) 또는 공간 곡선(space curve) 또는 표면(surfaces) 사이의 각도는 도함수(derivative)를 사용하여 계산될 수 있습니다.[54][55]

Curves

Main article: Curve (geometry)

곡선(curve)은 직선일 수도 있고 아닐 수도 있는 1-차원 대상입니다. 2-차원 공간에서 곡선은 평면 곡선(plane curve)이라고 불리고 3-차원 공간에서 그것들은 공간 곡선(space curve)이라고 불립니다.[56]

토폴로지에서, 곡선은 실수의 구간에서 또 다른 공간으로의 함수에 의해 정의됩니다.[49] 미분 기하학에서, 같은 정의가 사용되지만, 정의하는 함수는 미분-가능임을 요구됩니다. 대수적 기하학은 차원(dimension) 일의 대수적 다양체(algebraic varieties)로 정의되는 대수적 곡선(algebraic curve)을 연구합니다.[57]

Surfaces

Main article: Surface (mathematics)
A sphere is a surface that can be defined parametrically (by x = r sin θ cos φ, y = r sin θ sin φ, z = r cos θ) or implicitly (by x2 + y2 + z2 − r2 = 0.)

표면(surface)은 구 또는 포물면체와 같은 이-차원 대상입니다.[58] 미분 기하학(differential geometry)과[59] 토폴로지(topology)에서,[49] 표면은 각각 미분-동형(diffeomorphism) 또는 위상-동형(homeomorphism)에 의해 조립되는 이차원 '패치' (또는 이웃(neighborhoods))에 의해 설명됩니다. 대수적 기하학에서, 표면은 다항 방정식(polynomial equation)에 의해 설명됩니다.[57]

Manifolds

Main article: Manifold

매니폴드(manifold)는 곡선과 표면의 개념의 일반화입니다. 토폴로지(topology)에서, 매니폴드는 모든 각 점이 유클리드 공간과 위상동형적(homeomorphic)인 이웃(neighborhood)을 가지는 토폴로지적 공간(topological space)입니다.[49] 미분 기하학(differential geometry)에서, 미분가능 매니폴드(differentiable manifold)는 각 이웃이 유클리드 공간과 미분-동형적(diffeomorphic)인 공간입니다.[59]

매니폴드는 일반 상대성(general relativity)과 끈 이론(string theory)을 포함하여 물리학에서 광범위하게 사용됩니다.[60]

Length, area, and volume

Main articles: Length, Area, and Volume
See also: Area § List of formulas, and Volume § Volume formulas

길이(length), 넓이(area), 및 부피(volume)는 각각 일-차원, 이-차원, 및 삼-차원에서 대상의 크기 또는 범위를 설명합니다.[61]

유클리드 기하학(Euclidean geometry)과 해석적 기하학(analytic geometry)에서, 선분의 길이는 종종 피타고라스 정리(Pythagorean theorem)에 의해 계산될 수 있습니다.[62]

넓이와 부피는 길이와 별개의 기본 양으로 정의될 수 있거나, 그것들은 평면 또는 3-차원 공간에서 길이의 관점에서 설명되고 계산될 수 있습니다.[61] 수학자들은 다양한 기하학적 대상의 많은 명백한 넓이에 대한 공식과 부피에 대한 공식을 발견해 왔습니다. 미적분학(calculus)에서, 넓이와 부피는 리만 적분(Riemann integral)[63] 또는 르베그 적분(Lebesgue integral)과 같은 적분(integral)의 관점에서 정의될 수 있습니다.[64]

Metrics and measures

Main articles: Metric (mathematics) and Measure (mathematics)
Visual checking of the Pythagorean theorem for the (3, 4, 5) triangle as in the Zhoubi Suanjing 500–200 BC. The Pythagorean theorem is a consequence of the Euclidean metric.

길이 또는 거리의 개념은 일반화되고, 메트릭(metrics)의 아이디어로 이어질 수 있습니다.[65] 예를 들어, 유클리드 메트릭(Euclidean metric)은 유클리드 평면(Euclidean plane)에서 점 사이의 거리를 측정하고, 반면에 쌍곡선 메트릭(hyperbolic metric)은 쌍곡선 평면(hyperbolic plane)에서 거리를 측정합니다. 메트릭의 다른 중요한 예제는 특수 상대성(special relativity)의 로렌츠 메트릭(Lorentz metric)과 일반 상대성(general relativity)의 반-리만 메트릭(Riemannian metric)을 포함합니다.[66]

다른 방향에서, 길이, 넓이, 및 부피의 개념은 측정 이론(measure theory)에 의해 확장되며, 측정 이론은 크기 또는 측정을 집합(sets)에 할당하는 방법을 연구하며, 여기서 측정은 고전적 넓이와 부피의 규칙과 유사한 규칙을 따릅니다.[67]

Congruence and similarity

Main articles: Congruence (geometry) and Similarity (geometry)

합동(congruence)과 닮음(similarity)은 두 모양이 유사한 특성을 가질 때를 설명하는 개념입니다.[68] 유클리드 기하학에서, 닮음은 같은 모양을 가지는 대상을 설명하기 위해 사용되고, 반면에 합동은 크기와 모양 둘 다에서 같은 대상을 설명하기 위해 사용됩니다.[69] 힐베르트(Hilbert)는, 기하학에 대해 보다 엄격한 기초를 만드는 연구에서, 합동을 그것의 속성이 공리(axiom)에 의해 정의되는 정의되지 않은 용어로 취급했습니다.

합동과 닮음은 다양한 변환에 의해 보존되는 기하학적 대상의 속성을 연구하는 변환 기하학(transformation geometry)에서 일반화됩니다.[70]

Compass and straightedge constructions

Main article: Compass and straightedge constructions

고전 기하학자들은 일부 다른 방법에서 기술되어 왔던 기하학적 대상을 구성하기 위해 특별한 주의를 기울였습니다. 고전적으로, 대부분의 기하학적 구조에 사용되는 유일한 도구는 컴퍼스(compass)와 직선자(straightedge)뿐입니다.[b] 역시 모든 각 구조는 유한 단계의 숫자를 거쳐 완료되어야 했습니다. 어쨌든, 일부 문제는 이들 수단 단독에 의해 해결하기 어렵거나 불가능한 것으로 밝혀졌었고, 뉴시스(neusis), 포물선과 다른 곡선, 또는 기계적 장치를 사용하여 독창적인 구성이 발견되었습니다.

Dimension

Main article: Dimension
The Koch snowflake, with fractal dimension=log4/log3 and topological dimension=1

전통적인 기하학이 차원 1 (직선(line)), 2 (평면(plane)), 및 3 (삼-차원 공간(three-dimensional space)으로 여겨지는 우리의 주변 세계)을 허용하는 곳에, 수학자와 물리학자는 거의 2세기 동안 더 높은 차원(higher dimension)을 사용해 왔습니다.[71] 더 높은 차원에 대해 수학적 사용의 한 예제는 시스템의 자유도(degrees of freedom)와 같은 차원을 가지는 물리적 시스템의 구성 공간(configuration space)입니다. 예를 들어, 나사의 구성은 다섯의 좌표에 의해 설명될 수 있습니다.[72]

일반 토폴로지(general topology)에서, 차원의 개념은 자연수(natural number)에서 무한 차원 (예를 들어, 힐베르트 공간(Hilbert space))과 양의 실수(real number) (프랙탈 기하학(fractal geometry))로 확장되어 왔습니다.[73] 대수적 기하학(algebraic geometry)에서, 대수적 다양체의 차원(dimension of an algebraic variety)은 가장 공통적인 경우에 모두 동등한 여러 가지 명백하게 다른 정의를 받아 왔습니다.[74]

Symmetry

Main article: Symmetry
A tiling of the hyperbolic plane

기하학에서 대칭(symmetry)이라는 주제는 거의 기하학 자체의 과학만큼 오래되었습니다.[75] 원(circle), 정규 다각형(regular polygon), 플라톤 고체(platonic solid)와 같은 대칭적 모양은 많은 고대 철학자들에게 깊은 의미를 부여했었고[76] 유클리드 시대 이전에 자세하게 조사되었습니다.[39] 대칭적 패턴은 자연에서 발생하고 레오나르도 다 빈치(Leonardo da Vinci), 마우리츠 코르넬리스 에셔(M. C. Escher), 등의 그래픽을 포함하여 다양한 형식에서 예술적으로 표현되었습니다.[77] 19세기 후반에, 대칭과 기하학 사이의 관계가 집중적으로 조사되었습니다. 펠릭스 클라인(Felix Klein)의 에르랑겐 프로그램(Erlangen program)은, 매우 정확한 의미에서, 변환 그룹(group)의 개념을 통해 표현된 대칭이 기하학이 무엇인지를 결정한다고 선언했습니다.[78] 고전 유클리드 기하학(Euclidean geometry)에서 대칭은 합동(congruences)과 강체 운동에 의해 표현되고, 반면에 투영 기하학(projective geometry)에서 유사한 역할이 직선을 직선으로 바꾸는 기하학적 변형(geometric transformation), 공선형화(collineation)에 의해 수행됩니다.[79] 어쨌든, 그것은 '그것의 대칭 그룹(symmetry group)을 통해 기하학을 정의'하려는 클레인의 아이디어가 그것의 영감을 설립했던 보여이와 로바쳅스키, 리만, 클리퍼드(Clifford)와 클레인, 및 소푸스 리(Sophus Lie)의 새로운 기하학에서 였습니다.[80] 이산 대칭과 연속 대칭 둘 다는 기하학에서 중요한 역할을 하며, 전자는 토폴로지(topology)와 기하학 그룹 이론(geometric group theory)에서,[81][82] 후자는 리 이론(Lie theory)과 리만 기하학(Riemannian geometry)에서 중요한 역할을 합니다.[83][84]

다른 유형의 대칭은 다른 분야 중에서 투영 기하학(projective geometry)에서 이중성(duality)의 원리입니다. 이 메타-현상은 대략 다음과 같이 설명될 수 있습니다: 임의의 정리(theorem)에서, 점(point)과 평면(plane)을 교환하고, 결합하다(join)와 만나다(meet)를 교환하고, 놓여 있는(lies in)과 포함하다(contains)를 교환하고, 그 결과는 같게 참 정리입니다.[85] 유사하고 밀접하게 관련된 형식의 이중성이 벡터 공간(vector space)과 그것의 이중 공간(dual space) 사이에 존재합니다.[86]

Contemporary geometry

Euclidean geometry

Main article: Euclidean geometry

유클리드 기하학(Euclidean geometry)은 그것의 고전적 의미에서 기하학입니다.[87] 그것은 물리적 세계의 공간을 모델링하므로, 그것은 역학(mechanics), 천문학(astronomy), 결정학(crystallography)과 같은 많은 과학 분야와[88] 공학(engineering),[89] 건축(architecture),[90] 측지학(geodesy),[91] 공기역학(aerodynamics),[92] 및 항법(navigation)과 같은 많은 기술 분야에서 사용됩니다.[93] 대다수 국가의 의무 교육 커리큘럼은 점(points), 직선(lines), 평면(planes), 각도(angle), 삼각형(triangle), 합동(congruence), 닮음(similarity), 고체 도형(solid figure), 원(circle), 및 해석적 기하학(analytic geometry)과 같은 유클리드 개념의 연구를 포함합니다.[35]

Differential geometry

Differential geometry uses tools from calculus to study problems involving curvature.
Main article: Differential geometry

미분 기하학(differential geometry)은 기하학에서 문제를 연구하기 위해 미적분학(calculus)과 선형 대수(linear algebra)의 기술을 사용합니다.[94] 그것은 다른 것 중에서 물리학(physics),[95] 계량경제학(econometrics),[96] 생물정보학(bioinformatics)에[97] 응용을 가집니다.

특히, 미분 기하학은 우주(universe)가 구부러져(curved) 있다는 알베르트 아인슈타인(Albert Einstein)의 일반 상대성(general relativity) 공준으로 인해 수학적 물리학(mathematical physics)에서 중요합니다.[98] 미분 기하학은 내재적(intrinsic: 공간이 각 점 근처에서 거리가 측정되는 방법을 결정하는 그것의 기하학적 구조가 리만 메트릭(Riemannian metric)에 의해 지배되는 매끄러운 매니폴드(smooth manifold)임을 의미) 또는 외부적(extrinsic: 연구 중인 대상이 일부 주변 평평한 유클리드 공간의 일부인 곳)일 수 있습니다.[99]

Non-Euclidean geometry

Main article: Non-Euclidean geometry

유클리드 기하학은 연구된 기하학의 유일한 역사적 형식은 아니었습니다. 구형 기하학(spherical geometry)은 천문학자, 점성가, 및 항해사에 의해 오랫동안 사용되어 왔습니다.[100]

임마누엘 칸트(Immanuel Kant)는 마음의 내적 능력에 의해 선험(a priori)으로 참으로 알려져 있는 오직 하나의, 절대, 기하학이 있다고 주장했습니다: 유클리드 기하학은 합성적 선험(synthetic a priori)이었습니다.[101] 이 견해는 처음에 사케리(Saccheri)와 같은 사상가들에 의해 다소 도전을 받았고, 그런-다음 마침내 보여이, 로바쳅스키, 및 가우스 (그는 자신의 이론을 출판한 적이 없음)의 연구에서 비-유클리드 기하학(non-Euclidean geometry)의 혁명적인 발견에 의해 뒤집어졌니다.[102] 그들은 보통의 유클리드 공간(Euclidean space)이 기하학의 발전을 위한 단지 하나의 가능성일 뿐임을 보여주었습니다. 기하학의 주제에 대한 폭넓은 시각은 리만(Riemann)이 사망한 후에야 유일하게 출판된 그의 1867년 취임 강연 Über die Hypothesen, welche der Geometrie zu Grunde liegen (기하학의 기반이 되는 가설에 관하여)에서 표현되었습니다.[103] 공간의 리만의 새로운 아이디어는 알베르트 아인슈타인(Albert Einstein)의 일반 상대성 이론(general relativity theory)에서 결정적으로 입증되었습니다. 길이의 개념이 정의되는 매우 일반적인 공간을 고려하는 리만 기하학(Riemannian geometry)은 현대 기하학의 주류입니다.[80]

Topology

Main article: Topology
A thickening of the trefoil knot

토폴로지(topology)는 연속 매핑(continuous mapping)의 속성과 관련된 분야이고,[104] 유클리드 기하학의 일반화로 고려될 수 있습니다.[105] 실제에서, 토폴로지는 종종 연결성(connectedness) 및 컴팩트성(compactness)과 같은 공간의 대규모 속성을 다루는 것을 의미합니다.[49]

20세기에 엄청난 발전을 보인 토폴로지 분야는 기술적인 의미에서 변환이 위상-동형(homeomorphism)인 변환 기하학(transformation geometry)의 한 유형입니다.[106] 이것은 종종 '토폴로지는 고무-시트 기하학이다'라고 말하는 것의 형식에서 표현되어 왔습니다. 토폴로지의 부분필드는 기하학적 토폴로지(geometric topology), 미분 토폴로지(differential topology), 대수적 토폴로지(algebraic topology), 및 일반 토폴로지(general topology)를 포함합니다.[107]

Algebraic geometry

Main article: Algebraic geometry
Quintic Calabi–Yau threefold

대수적 기하학(algebraic geometry)의 분야는 좌표(co-ordinates)의 데카르트 기하학(Cartesian geometry)에서 발전되었습니다.[108] 그것은 다른 주제 중에서 투영 기하학(projective geometry), 쌍유리 기하학(birational geometry), 대수적 다양체(algebraic varieties), 및 교환 대수학(commutative algebra)의 생성과 연구와 함께 성장의 주기적인 기간을 거쳤습니다.[109] 1950년대 후반부터 1970년대 중반동안 내내, 그것은 장-피에르 세르(Jean-Pierre Serre)와 알렉산더 그로텐디크(Alexander Grothendieck)의 연구로 인해 주요 기초 개발이 이루어졌습니다.[109] 이것은 다양한 코호몰로지 이론(cohomology theories)을 포함한 스킴(schemes)의 도입과 토폴로지적(topological) 방법에 대한 더 큰 강조로 이어졌습니다. 일곱 밀레니엄 상 문제(Millennium Prize Problems) 중 하나, 호지 추측(Hodge conjecture)은 대수적 기하학의 하나의 문제입니다.[110] 페르마의 마지막 정리의 와일스의 증명(Wiles' proof of Fermat's Last Theorem)은 숫자 이론(number theory)의 오래-지속된 문제를 해결하기 위해 고급 대수적 기하학의 방법을 사용합니다.

일반적으로, 대수적 기하학은 다변수 다항식(multivariate polynomial)과 같은 교환 대수학(commutative algebra)의 개념의 사용을 통해 기하학을 연구합니다.[111] 그것은 암호화(cryptography)와[112] 끈 이론(string theory)을 포함한 많은 영역에서 응용을 가집니다.[113]

Complex geometry

Main article: Complex geometry

복소 기하학(complex geometry)은 복소 평면(complex plane)에서 모델링되거나, 복소 평면에서 발생하는 기하학적 구조의 본성을 연구합니다.[114][115][116] 복소 기하학은 미분 기하학, 대수적 기하학, 및 여러 복소 변수(several complex variables)의 해석학의 교차에 놓여있고, 끈 이론(string theory)과 거울 대칭(mirror symmetry)에 대한 응용을 발견해 왔습니다.[117]

복소 기하학은 베른하르트 리만(Bernhard Riemann)의 리만 표면(Riemann surface)의 그의 연구에서 독특한 연구의 영역으로 처음 등장했습니다.[118][119][120] 리만의 정신에 입각한 연구는 1900년대 초에 이탈리아의 대수 기하학 학교에서 수행되었습니다. 복소 기하학의 현대적 치료는 뭉치(sheaves)의 개념을 주제에 도입하고, 복소 기하학과 대수적 기하학 사이의 관계를 조명한 장-피에르 세르(Jean-Pierre Serre)의 연구로 시작되었습니다.[121][122] 복소 기하학에서 연구의 주요 대상은 복소 매니폴드(complex manifold), 복소 대수적 다양체(complex algebraic varieties), 복소 해석적 다양체(complex analytic varieties), 및 이들 공간에 걸쳐 정칙 벡터 다발(holomorphic vector bundles)과 일관된 뭉치(coherent sheaves)입니다. 복소 기하학에서 연구된 공간의 특별한 예제는 리만 표면, 및 칼라비–야우 매니폴드(Calabi–Yau manifold)를 포함하고, 이들 공간은 끈 이론에서 사용을 찾습니다. 특히, 끈의 세계시트(worldsheet)는 리만 표면에 의해 모델링되고, 초월끈 이론(superstring theory)은 10 차원 시공간(spacetime)의 여분의 6 차원이 칼라비–야우 매니폴드에 의해 모델링될 수 있다고 예측합니다.

Discrete geometry

Main article: Discrete geometry
Discrete geometry includes the study of various sphere packings.

이산 기하학(discrete geometry)은 볼록 기하학(convex geometry)과 밀접한 연결을 가지는 주제입니다.[123][124][125] 그것은 주로 점, 직선, 원과 같은 단순한 기하학적 대상의 상대적 위치의 질문과 관련이 있습니다. 예제는 구 패킹(sphere packing), 삼각분할(triangulations), 크네즈-포울센(Kneser-Poulsen) 추측, 등의 연구를 포함합니다.[126][127] 그것은 조합론(combinatorics)과 많은 방법과 원칙을 공유합니다.

Computational geometry

Main article: Computational geometry

계산 기하학(computational geometry)은 기하학적 대상을 조작하기 위한 알고리듬(algorithm)과 그것들의 구현(implementations)을 다룹니다. 역사적으로 중요한 문제는 여행하는 세일즈맨 문제(traveling salesman problem), 최소 스패닝 트리(minimum spanning tree), 숨긴-직선 제거(hidden-line removal), 및 선형 프로그래밍(linear programming)을 포함해 왔습니다.[128]

비록 기하학의 젊은 영역이지만, 그것은 컴퓨터 비전(computer vision), 이미지 처리(image processing), 컴퓨터-지원 설계(computer-aided design), 의료 이미징(medical imaging), 등에 많은 응용을 가집니다.[129]

Geometric group theory

Main article: Geometric group theory
The Cayley graph of the free group on two generators a and b

기하학적 그룹 이론(geometric group theory)은 대규모 기하학적 기술을 유한하게 생성된 그룹(finitely generated group)을 연구하기 위해 사용합니다.[130] 그것은 밀레니엄 상 문제(Millennium Prize Problem), 푸앵카레 추측(Poincaré conjecture)의 증명을 포함했던, 그리고리 페렐만(Grigori Perelman)의 기하학화 추측(Geometrization conjecture)의 증명과 같은 낮은-차원 토폴로지(low-dimensional topology)와 밀접하게 연결되어 있습니다.[131]

기하학적 그룹 이론은 종종 그룹의 기하학적 표현인 케일리 그래프(Cayley graph)를 중심으로 회전합니다. 다른 중요한 주제로는 준-등거리변환(quasi-isometries), 그로모프-쌍곡선 그룹(Gromov-hyperbolic group), 및 직각 아르틴 그룹(right angled Artin group)을 포함합니다.[130][132]

Convex geometry

Main article: Convex geometry

볼록 기하학(convex geometry)은 종종 실수 해석학(real analysis)과 이산 수학(discrete mathematics)의 기술을 사용하여 유클리드 공간에서 볼록(convex) 모양과 보다 추상적인 유사체를 조사합니다.[133] 그것은 볼록 해석학(convex analysis), 최적화(optimization), 및 함수형 해석학(functional analysis)과 숫자 이론(number theory)에서 중요한 응용과 밀접한 관련이 있습니다.

볼록 기하학은 고대로 거슬러 올라갑니다.[133] 아르키메데스(Archimedes)는 볼록성의 최초의 정확한 정의를 제공했습니다. 볼록 기하학에서 되풀이되는 개념, 같은-둘레 문제(isoperimetric problem)는 제노도로스(Zenodorus)를 비롯한 그리스인들도 연구했습니다. 아르키메데스, 플라톤(Plato), 유클리드(Euclid), 및 나중에 케플러(Kepler)와 콕세터(Coxeter) 모두는 볼록 폴리토프(convex polytope)와 그 속성을 연구했습니다. 19세기부터, 수학자들은 고차원 폴리토프, 볼록 몸체의 부피와 표면 넓이, 가우스 곡률(Gaussian curvature), 알고리듬(algorithms), 타일링(tilings)과 격자(lattice)를 포함하여 볼록 수학의 다른 영역을 연구해 왔습니다.

Applications

기하학은 다양한 분야에서 응용을 발견해 왔고, 그 중 일부는 아래에 설명되어 있습니다.

Art

Main article: Mathematics and art
Bou Inania Madrasa, Fes, Morocco, zellige mosaic tiles forming elaborate geometric tessellations

수학과 예술은 다양한 방법에서 관련되어 있습니다. 예를 들어, 원근법(perspective) 이론은 단지 도형의 메트릭 속성보다 기하학에 더 많은 것이 있음을 보여주었습니다: 원근법은 투영 기하학(projective geometry)의 기원입니다.[134]

예술가들은 오랫동안 디자인에서 비율(proportion)의 개념을 사용해 왔습니다. 비트루비우스(Vitruvius)는 인체의 이상적인 비율의 복잡한 이론을 발전시켰습니다.[135] 이들 개념은 미켈란젤로(Michelangelo)에서 현대 만화가에 이르기까지 예술가들에 의해 사용하고 적용되어 왔습니다.[136]

황금 비율(golden ratio)은 예술에서 논쟁의 여지를 가져왔던 특정 비율입니다. 가장 미학적으로 아름다운 길이의 비율이라고 주장되는 경우가 종종 있지만, 가장 신뢰할 수 있고 명확한 예제는 이 전설을 알고 있는 예술가에 의해 의도적으로 만들어진 것이긴 하지만 유명한 예술 작품에 통합되는 경우가 많습니다.[137]

타일링(tilings), 또는 테셀레이션은 역사를 통틀어 예술에서 사용되어 왔습니다. 이슬람 예술(Islamic art)은 마우리츠 코르넬리스 에셔(M. C. Escher)의 예술과 마찬가지로 테셀레이션을 자주 사용합니다.[138] 에셔의 작품은 역시 쌍곡선 기하학(hyperbolic geometry)을 사용했습니다.

세잔(Cézanne)은 모든 이미지가 구(sphere), 원뿔(cone), 및 원기둥(cylinder)에서 만들어질 수 있다는 이론을 발전시켰습니다. 비록 정확한 모양의 목록은 저자마다 다르지만, 이것은 오늘날에도 여전히 예술 이론에서 사용됩니다.[139][140]

Architecture

Main articles: Mathematics and architecture and Architectural geometry

기하학은 건축에서 많은 응용을 가집니다. 사실, 기하학은 건축 디자인의 핵심에 놓여 있다고 말해져 왔습니다.[141][142] 건축에 대한 기하학의 응용은 강제된 원근법(forced perspective)을 생성하기 위한 투영 기하학(projective geometry)의 사용,[143] 돔과 유사한 물체를 구성하는 것에서 원뿔 단면(conic section)의 사용,[90] 테셀레이션(tessellations)의 사용,[90] 대칭의 사용을 포함합니다.[90]

Physics

Main article: Mathematical physics

천문학(astronomy)의 분야는, 특히 천구(celestial sphere) 위에 별(star)과 행성(planet)의 위치를 매핑하고 천체의 움직임 사이의 관계를 설명하는 것과 관련하여, 역사 전반에 걸쳐 기하학적 문제의 중요한 원천으로 사용되어 왔습니다.[144]

리만 기하학(Riemannian geometry)과 유사-리만(pseudo-Riemannian) 기하학이 일반 상대성(general relativity)에서 사용됩니다.[145] 끈 이론(string theory)은 양자 정보 이론(quantum information theory)과 마찬가지로[146] 기하학의 여러 변형을 사용합니다.[147]

Other fields of mathematics

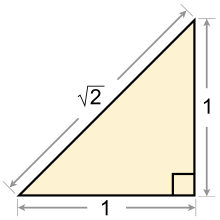
The Pythagoreans discovered that the sides of a triangle could have incommensurable lengths.

미적분학(Calculus)은 기하학의 영향을 강하게 받았습니다.[29] 예를 들어, 르네 데카르트(René Descartes)에 의한 좌표(coordinates)의 도입과 대수학의 동시 발전은 기하학에 대해 새로운 단계를 표시했는데, 왜냐하면 평면 곡선(plane curve)과 같은 기하학적 도형이 이제 함수와 방정식의 형식에서 해석적(analytically)으로 표현될 수 있기 때문입니다. 이것은 17세기에서 무한소 미적분학(infinitesimal calculus)의 출현에 중요한 역할을 했습니다. 해석적 기하학은 미적분학-이전 과정과 미적분학 교과과정에서 계속해서 주류를 이루고 있습니다.[148][149]

응용의 또 다른 중요한 영역은 숫자 이론(number theory)입니다.[150] 고대 그리스(ancient Greece)에서, 피타고라스 학파(Pythagoreans)는 기하학에서 숫자의 역할을 고려했습니다. 어쨌든, 비-정수비율가능 길이의 발견은 그들의 철학적 견해와 모순되었습니다.[151] 19세기이후, 기하학은, 예를 들어, 숫자의 기하학(geometry of numbers)을 통해, 또는 보다 최근에는 페르마의 마지막 정리의 와일스의 증명(Wiles's proof of Fermat's Last Theorem)에 사용되는 스킴 이론을 통해 스킴 이론(scheme theory)에서 문제를 해결하는 데 사용되어 왔습니다.[152]

See also

Lists

Related topics

Other fields

Notes

  1. ^ Until the 19th century, geometry was dominated by the assumption that all geometric constructions were Euclidean. In the 19th century and later, this was challenged by the development of hyperbolic geometry by Lobachevsky and other non-Euclidean geometries by Gauss and others. It was then realised that implicitly non-Euclidean geometry had appeared throughout history, including the work of Desargues in the 17th century, all the way back to the implicit use of spherical geometry to understand the Earth geodesy and to navigate the oceans since antiquity.
  2. ^ The ancient Greeks had some constructions using other instruments.
  1. ^ Vincenzo De Risi (2015). Mathematizing Space: The Objects of Geometry from Antiquity to the Early Modern Age. Birkhäuser. pp. 1–. ISBN 978-3-319-12102-4.
  2. ^ a b Tabak, John (2014). Geometry: the language of space and form. Infobase Publishing. p. xiv. ISBN 978-0-8160-4953-0.
  3. ^ Walter A. Meyer (2006). Geometry and Its Applications. Elsevier. ISBN 978-0-08-047803-6.
  4. ^ J. Friberg, "Methods and traditions of Babylonian mathematics. Plimpton 322, Pythagorean triples, and the Babylonian triangle parameter equations", Historia Mathematica, 8, 1981, pp. 277–318.
  5. ^ Neugebauer, Otto (1969) [1957]. "Chap. IV Egyptian Mathematics and Astronomy". The Exact Sciences in Antiquity (2 ed.). Dover Publications. pp. 71–96. ISBN 978-0-486-22332-2..
  6. ^ (Boyer 1991, "Egypt" p. 19)
  7. ^ Ossendrijver, Mathieu (29 January 2016). "Ancient Babylonian astronomers calculated Jupiter's position from the area under a time-velocity graph". Science. 351 (6272): 482–484. Bibcode:2016Sci...351..482O. doi:10.1126/science.aad8085. PMID 26823423. S2CID 206644971.
  8. ^ Depuydt, Leo (1 January 1998). "Gnomons at Meroë and Early Trigonometry". The Journal of Egyptian Archaeology. 84: 171–180. doi:10.2307/3822211. JSTOR 3822211.
  9. ^ Slayman, Andrew (27 May 1998). "Neolithic Skywatchers". Archaeology Magazine Archive. Archived from the original on 5 June 2011. Retrieved 17 April 2011.
  10. ^ (Boyer 1991, "Ionia and the Pythagoreans" p. 43)
  11. ^ Eves, Howard, An Introduction to the History of Mathematics, Saunders, 1990, ISBN 0-03-029558-0.
  12. ^ Kurt Von Fritz (1945). "The Discovery of Incommensurability by Hippasus of Metapontum". The Annals of Mathematics.
  13. ^ James R. Choike (1980). "The Pentagram and the Discovery of an Irrational Number". The Two-Year College Mathematics Journal.
  14. ^ (Boyer 1991, "The Age of Plato and Aristotle" p. 92)
  15. ^ (Boyer 1991, "Euclid of Alexandria" p. 104)
  16. ^ Howard Eves, An Introduction to the History of Mathematics, Saunders, 1990, ISBN 0-03-029558-0 p. 141: "No work, except The Bible, has been more widely used...."
  17. ^ O'Connor, J.J.; Robertson, E.F. (February 1996). "A history of calculus". University of St Andrews. Archived from the original on 15 July 2007. Retrieved 7 August 2007.
  18. ^ Staal, Frits (1999). "Greek and Vedic Geometry". Journal of Indian Philosophy. 27 (1–2): 105–127. doi:10.1023/A:1004364417713. S2CID 170894641.
  19. ^ Pythagorean triples are triples of integers with the property: . Thus, , , etc.
  20. ^ (Cooke 2005, p. 198): "The arithmetic content of the Śulva Sūtras consists of rules for finding Pythagorean triples such as (3, 4, 5), (5, 12, 13), (8, 15, 17), and (12, 35, 37). It is not certain what practical use these arithmetic rules had. The best conjecture is that they were part of religious ritual. A Hindu home was required to have three fires burning at three different altars. The three altars were to be of different shapes, but all three were to have the same area. These conditions led to certain "Diophantine" problems, a particular case of which is the generation of Pythagorean triples, so as to make one square integer equal to the sum of two others."
  21. ^ (Hayashi 2005, p. 371)
  22. ^ a b (Hayashi 2003, pp. 121–122)
  23. ^ R. Rashed (1994), The development of Arabic mathematics: between arithmetic and algebra, p. 35 London
  24. ^ (Boyer 1991, "The Arabic Hegemony" pp. 241–242) "Omar Khayyam (c. 1050–1123), the "tent-maker," wrote an Algebra that went beyond that of al-Khwarizmi to include equations of third degree. Like his Arab predecessors, Omar Khayyam provided for quadratic equations both arithmetic and geometric solutions; for general cubic equations, he believed (mistakenly, as the 16th century later showed), arithmetic solutions were impossible; hence he gave only geometric solutions. The scheme of using intersecting conics to solve cubics had been used earlier by Menaechmus, Archimedes, and Alhazan, but Omar Khayyam took the praiseworthy step of generalizing the method to cover all third-degree equations (having positive roots). .. For equations of higher degree than three, Omar Khayyam evidently did not envision similar geometric methods, for space does not contain more than three dimensions, ... One of the most fruitful contributions of Arabic eclecticism was the tendency to close the gap between numerical and geometric algebra. The decisive step in this direction came much later with Descartes, but Omar Khayyam was moving in this direction when he wrote, "Whoever thinks algebra is a trick in obtaining unknowns has thought it in vain. No attention should be paid to the fact that algebra and geometry are different in appearance. Algebras are geometric facts which are proved."".
  25. ^ O'Connor, John J.; Robertson, Edmund F., "Al-Mahani", MacTutor History of Mathematics archive, University of St Andrews.
  26. ^ O'Connor, John J.; Robertson, Edmund F., "Al-Sabi Thabit ibn Qurra al-Harrani", MacTutor History of Mathematics archive, University of St Andrews.
  27. ^ O'Connor, John J.; Robertson, Edmund F., "Omar Khayyam", MacTutor History of Mathematics archive, University of St Andrews.
  28. ^ Boris A. Rosenfeld and Adolf P. Youschkevitch (1996), "Geometry", in Roshdi Rashed, ed., Encyclopedia of the History of Arabic Science, Vol. 2, pp. 447–494 [470], Routledge, London and New York:

    "Three scientists, Ibn al-Haytham, Khayyam, and al-Tusi, had made the most considerable contribution to this branch of geometry whose importance came to be completely recognized only in the 19th century. In essence, their propositions concerning the properties of quadrangles which they considered, assuming that some of the angles of these figures were acute of obtuse, embodied the first few theorems of the hyperbolic and the elliptic geometries. Their other proposals showed that various geometric statements were equivalent to the Euclidean postulate V. It is extremely important that these scholars established the mutual connection between this postulate and the sum of the angles of a triangle and a quadrangle. By their works on the theory of parallel lines Arab mathematicians directly influenced the relevant investigations of their European counterparts. The first European attempt to prove the postulate on parallel lines—made by Witelo, the Polish scientists of the 13th century, while revising Ibn al-Haytham's Book of Optics (Kitab al-Manazir)—was undoubtedly prompted by Arabic sources. The proofs put forward in the 14th century by the Jewish scholar Levi ben Gerson, who lived in southern France, and by the above-mentioned Alfonso from Spain directly border on Ibn al-Haytham's demonstration. Above, we have demonstrated that Pseudo-Tusi's Exposition of Euclid had stimulated both J. Wallis's and G. Saccheri's studies of the theory of parallel lines."
  29. ^ a b Carl B. Boyer (2012). History of Analytic Geometry. Courier Corporation. ISBN 978-0-486-15451-0.
  30. ^ C.H. Edwards Jr. (2012). The Historical Development of the Calculus. Springer Science & Business Media. p. 95. ISBN 978-1-4612-6230-5.
  31. ^ Judith V. Field; Jeremy Gray (2012). The Geometrical Work of Girard Desargues. Springer Science & Business Media. p. 43. ISBN 978-1-4613-8692-6.
  32. ^ C. R. Wylie (2011). Introduction to Projective Geometry. Courier Corporation. ISBN 978-0-486-14170-1.
  33. ^ Jeremy Gray (2011). Worlds Out of Nothing: A Course in the History of Geometry in the 19th Century. Springer Science & Business Media. ISBN 978-0-85729-060-1.
  34. ^ Eduardo Bayro-Corrochano (2018). Geometric Algebra Applications Vol. I: Computer Vision, Graphics and Neurocomputing. Springer. p. 4. ISBN 978-3-319-74830-6.
  35. ^ a b Schmidt, W., Houang, R., & Cogan, L. (2002). "A coherent curriculum". American Educator, 26(2), 1–18.
  36. ^ Morris Kline (1990). Mathematical Thought From Ancient to Modern Times: Volume 3. US: Oxford University Press. pp. 1010–. ISBN 978-0-19-506137-6.
  37. ^ Victor J. Katz (2000). Using History to Teach Mathematics: An International Perspective. Cambridge University Press. pp. 45–. ISBN 978-0-88385-163-0.
  38. ^ David Berlinski (2014). The King of Infinite Space: Euclid and His Elements. Basic Books. ISBN 978-0-465-03863-3.
  39. ^ a b Robin Hartshorne (2013). Geometry: Euclid and Beyond. Springer Science & Business Media. pp. 29–. ISBN 978-0-387-22676-7.
  40. ^ Pat Herbst; Taro Fujita; Stefan Halverscheid; Michael Weiss (2017). The Learning and Teaching of Geometry in Secondary Schools: A Modeling Perspective. Taylor & Francis. pp. 20–. ISBN 978-1-351-97353-3.
  41. ^ I.M. Yaglom (2012). A Simple Non-Euclidean Geometry and Its Physical Basis: An Elementary Account of Galilean Geometry and the Galilean Principle of Relativity. Springer Science & Business Media. pp. 6–. ISBN 978-1-4612-6135-3.
  42. ^ Audun Holme (2010). Geometry: Our Cultural Heritage. Springer Science & Business Media. pp. 254–. ISBN 978-3-642-14441-7.
  43. ^ a b c d e Euclid's Elements – All thirteen books in one volume, Based on Heath's translation, Green Lion Press ISBN 1-888009-18-7.
  44. ^ Gerla, G. (1995). "Pointless Geometries" (PDF). In Buekenhout, F.; Kantor, W. (eds.). Handbook of incidence geometry: buildings and foundations. North-Holland. pp. 1015–1031. Archived from the original (PDF) on 17 July 2011.
  45. ^ Clark, Bowman L. (Jan 1985). "Individuals and Points". Notre Dame Journal of Formal Logic. 26 (1): 61–75. doi:10.1305/ndjfl/1093870761.
  46. ^ John Casey (1885). Analytic Geometry of the Point, Line, Circle, and Conic Sections.
  47. ^ Buekenhout, Francis (1995), Handbook of Incidence Geometry: Buildings and Foundations, Elsevier B.V.
  48. ^ "geodesic – definition of geodesic in English from the Oxford dictionary". OxfordDictionaries.com. Archived from the original on 15 July 2016. Retrieved 2016-01-20.
  49. ^ a b c d e Munkres, James R. Topology. Vol. 2. Upper Saddle River: Prentice Hall, 2000.
  50. ^ Szmielew, Wanda. From affine to Euclidean geometry: An axiomatic approach. Springer, 1983.
  51. ^ Ahlfors, Lars V. Complex analysis: an introduction to the theory of analytic functions of one complex variable. New York; London (1953).
  52. ^ Sidorov, L.A. (2001) [1994]. "Angle". Encyclopedia of Mathematics. EMS Press.
  53. ^ Gelʹfand, Izrailʹ Moiseevič, and Mark Saul. "Trigonometry." Trigonometry. Birkhäuser Boston, 2001. 1–20.
  54. ^ Stewart, James (2012). Calculus: Early Transcendentals, 7th ed., Brooks Cole Cengage Learning. ISBN 978-0-538-49790-9
  55. ^ Jost, Jürgen (2002). Riemannian Geometry and Geometric Analysis. Berlin: Springer-Verlag. ISBN 978-3-540-42627-1..
  56. ^ Baker, Henry Frederick. Principles of geometry. Vol. 2. CUP Archive, 1954.
  57. ^ a b Mumford, David (1999). The Red Book of Varieties and Schemes Includes the Michigan Lectures on Curves and Their Jacobians (2nd ed.). Springer-Verlag. ISBN 978-3-540-63293-1. Zbl 0945.14001.
  58. ^ Briggs, William L., and Lyle Cochran Calculus. "Early Transcendentals." ISBN 978-0-321-57056-7.
  59. ^ a b Do Carmo, Manfredo Perdigao, and Manfredo Perdigao Do Carmo. Differential geometry of curves and surfaces. Vol. 2. Englewood Cliffs: Prentice-hall, 1976.
  60. ^ Yau, Shing-Tung; Nadis, Steve (2010). The Shape of Inner Space: String Theory and the Geometry of the Universe's Hidden Dimensions. Basic Books. ISBN 978-0-465-02023-2.
  61. ^ a b Steven A. Treese (2018). History and Measurement of the Base and Derived Units. Springer International Publishing. pp. 101–. ISBN 978-3-319-77577-7.
  62. ^ James W. Cannon (2017). Geometry of Lengths, Areas, and Volumes. American Mathematical Soc. p. 11. ISBN 978-1-4704-3714-5.
  63. ^ Gilbert Strang (1991). Calculus. SIAM. ISBN 978-0-9614088-2-4.
  64. ^ H. S. Bear (2002). A Primer of Lebesgue Integration. Academic Press. ISBN 978-0-12-083971-1.
  65. ^ Dmitri Burago, Yu D Burago, Sergei Ivanov, A Course in Metric Geometry, American Mathematical Society, 2001, ISBN 0-8218-2129-6.
  66. ^ Wald, Robert M. (1984). General Relativity. University of Chicago Press. ISBN 978-0-226-87033-5.
  67. ^ Terence Tao (2011). An Introduction to Measure Theory. American Mathematical Soc. ISBN 978-0-8218-6919-2.
  68. ^ Shlomo Libeskind (2008). Euclidean and Transformational Geometry: A Deductive Inquiry. Jones & Bartlett Learning. p. 255. ISBN 978-0-7637-4366-6.
  69. ^ Mark A. Freitag (2013). Mathematics for Elementary School Teachers: A Process Approach. Cengage Learning. p. 614. ISBN 978-0-618-61008-2.
  70. ^ George E. Martin (2012). Transformation Geometry: An Introduction to Symmetry. Springer Science & Business Media. ISBN 978-1-4612-5680-9.
  71. ^ Mark Blacklock (2018). The Emergence of the Fourth Dimension: Higher Spatial Thinking in the Fin de Siècle. Oxford University Press. ISBN 978-0-19-875548-7.
  72. ^ Charles Jasper Joly (1895). Papers. The Academy. pp. 62–.
  73. ^ Roger Temam (2013). Infinite-Dimensional Dynamical Systems in Mechanics and Physics. Springer Science & Business Media. p. 367. ISBN 978-1-4612-0645-3.
  74. ^ Bill Jacob; Tsit-Yuen Lam (1994). Recent Advances in Real Algebraic Geometry and Quadratic Forms: Proceedings of the RAGSQUAD Year, Berkeley, 1990–1991. American Mathematical Soc. p. 111. ISBN 978-0-8218-5154-8.
  75. ^ Ian Stewart (2008). Why Beauty Is Truth: A History of Symmetry. Basic Books. p. 14. ISBN 978-0-465-08237-7.
  76. ^ Stakhov Alexey (2009). Mathematics Of Harmony: From Euclid To Contemporary Mathematics And Computer Science. World Scientific. p. 144. ISBN 978-981-4472-57-9.
  77. ^ Werner Hahn (1998). Symmetry as a Developmental Principle in Nature and Art. World Scientific. ISBN 978-981-02-2363-2.
  78. ^ Brian J. Cantwell (2002). Introduction to Symmetry Analysis. Cambridge University Press. p. 34. ISBN 978-1-139-43171-2.
  79. ^ B. Rosenfeld; Bill Wiebe (2013). Geometry of Lie Groups. Springer Science & Business Media. pp. 158ff. ISBN 978-1-4757-5325-7.
  80. ^ a b Peter Pesic (2007). Beyond Geometry: Classic Papers from Riemann to Einstein. Courier Corporation. ISBN 978-0-486-45350-7.
  81. ^ Michio Kaku (2012). Strings, Conformal Fields, and Topology: An Introduction. Springer Science & Business Media. p. 151. ISBN 978-1-4684-0397-8.
  82. ^ Mladen Bestvina; Michah Sageev; Karen Vogtmann (2014). Geometric Group Theory. American Mathematical Soc. p. 132. ISBN 978-1-4704-1227-2.
  83. ^ W-H. Steeb (1996). Continuous Symmetries, Lie Algebras, Differential Equations and Computer Algebra. World Scientific Publishing Company. ISBN 978-981-310-503-4.
  84. ^ Charles W. Misner (2005). Directions in General Relativity: Volume 1: Proceedings of the 1993 International Symposium, Maryland: Papers in Honor of Charles Misner. Cambridge University Press. p. 272. ISBN 978-0-521-02139-5.
  85. ^ Linnaeus Wayland Dowling (1917). Projective Geometry. McGraw-Hill book Company, Incorporated. p. 10.
  86. ^ G. Gierz (2006). Bundles of Topological Vector Spaces and Their Duality. Springer. p. 252. ISBN 978-3-540-39437-2.
  87. ^ Robert E. Butts; J.R. Brown (2012). Constructivism and Science: Essays in Recent German Philosophy. Springer Science & Business Media. pp. 127–. ISBN 978-94-009-0959-5.
  88. ^ Science. Moses King. 1886. pp. 181–.
  89. ^ W. Abbot (2013). Practical Geometry and Engineering Graphics: A Textbook for Engineering and Other Students. Springer Science & Business Media. pp. 6–. ISBN 978-94-017-2742-6.
  90. ^ a b c d George L. Hersey (2001). Architecture and Geometry in the Age of the Baroque. University of Chicago Press. ISBN 978-0-226-32783-9.
  91. ^ P. Vanícek; E.J. Krakiwsky (2015). Geodesy: The Concepts. Elsevier. p. 23. ISBN 978-1-4832-9079-9.
  92. ^ Russell M. Cummings; Scott A. Morton; William H. Mason; David R. McDaniel (2015). Applied Computational Aerodynamics. Cambridge University Press. p. 449. ISBN 978-1-107-05374-8.
  93. ^ Roy Williams (1998). Geometry of Navigation. Horwood Pub. ISBN 978-1-898563-46-4.
  94. ^ Gerard Walschap (2015). Multivariable Calculus and Differential Geometry. De Gruyter. ISBN 978-3-11-036954-0.
  95. ^ Harley Flanders (2012). Differential Forms with Applications to the Physical Sciences. Courier Corporation. ISBN 978-0-486-13961-6.
  96. ^ Paul Marriott; Mark Salmon (2000). Applications of Differential Geometry to Econometrics. Cambridge University Press. ISBN 978-0-521-65116-5.
  97. ^ Matthew He; Sergey Petoukhov (2011). Mathematics of Bioinformatics: Theory, Methods and Applications. John Wiley & Sons. p. 106. ISBN 978-1-118-09952-0.
  98. ^ P.A.M. Dirac (2016). General Theory of Relativity. Princeton University Press. ISBN 978-1-4008-8419-3.
  99. ^ Nihat Ay; Jürgen Jost; Hông Vân Lê; Lorenz Schwachhöfer (2017). Information Geometry. Springer. p. 185. ISBN 978-3-319-56478-4.
  100. ^ Boris A. Rosenfeld (2012). A History of Non-Euclidean Geometry: Evolution of the Concept of a Geometric Space. Springer Science & Business Media. ISBN 978-1-4419-8680-1.
  101. ^ Kline (1972) "Mathematical thought from ancient to modern times", Oxford University Press, p. 1032. Kant did not reject the logical (analytic a priori) possibility of non-Euclidean geometry, see Jeremy Gray, "Ideas of Space Euclidean, Non-Euclidean, and Relativistic", Oxford, 1989; p. 85. Some have implied that, in light of this, Kant had in fact predicted the development of non-Euclidean geometry, cf. Leonard Nelson, "Philosophy and Axiomatics," Socratic Method and Critical Philosoph, Dover, 1965, p. 164.
  102. ^ Duncan M'Laren Young Sommerville (1919). Elements of Non-Euclidean Geometry ... Open Court. pp. 15ff.
  103. ^ "Ueber die Hypothesen, welche der Geometrie zu Grunde liegen". Archived from the original on 18 March 2016.
  104. ^ Martin D. Crossley (2011). Essential Topology. Springer Science & Business Media. ISBN 978-1-85233-782-7.
  105. ^ Charles Nash; Siddhartha Sen (1988). Topology and Geometry for Physicists. Elsevier. p. 1. ISBN 978-0-08-057085-3.
  106. ^ George E. Martin (1996). Transformation Geometry: An Introduction to Symmetry. Springer Science & Business Media. ISBN 978-0-387-90636-2.
  107. ^ J. P. May (1999). A Concise Course in Algebraic Topology. University of Chicago Press. ISBN 978-0-226-51183-2.
  108. ^ The Encyclopedia Americana: A Universal Reference Library Comprising the Arts and Sciences, Literature, History, Biography, Geography, Commerce, Etc., of the World. Scientific American Compiling Department. 1905. pp. 489–.
  109. ^ a b Suzanne C. Dieudonne (1985). History Algebraic Geometry. CRC Press. ISBN 978-0-412-99371-8.
  110. ^ James Carlson; James A. Carlson; Arthur Jaffe; Andrew Wiles (2006). The Millennium Prize Problems. American Mathematical Soc. ISBN 978-0-8218-3679-8.
  111. ^ Robin Hartshorne (2013). Algebraic Geometry. Springer Science & Business Media. ISBN 978-1-4757-3849-0.
  112. ^ Everett W. Howe; Kristin E. Lauter; Judy L. Walker (2017). Algebraic Geometry for Coding Theory and Cryptography: IPAM, Los Angeles, CA, February 2016. Springer. ISBN 978-3-319-63931-4.
  113. ^ Marcos Marino; Michael Thaddeus; Ravi Vakil (2008). Enumerative Invariants in Algebraic Geometry and String Theory: Lectures given at the C.I.M.E. Summer School held in Cetraro, Italy, June 6–11, 2005. Springer. ISBN 978-3-540-79814-9.
  114. ^ Huybrechts, D. (2006). Complex geometry: an introduction. Springer Science & Business Media.
  115. ^ Griffiths, P., & Harris, J. (2014). Principles of algebraic geometry. John Wiley & Sons.
  116. ^ Wells, R. O. N., & García-Prada, O. (1980). Differential analysis on complex manifolds (Vol. 21980). New York: Springer.
  117. ^ Hori, K., Thomas, R., Katz, S., Vafa, C., Pandharipande, R., Klemm, A., ... & Zaslow, E. (2003). Mirror symmetry (Vol. 1). American Mathematical Soc.
  118. ^ Forster, O. (2012). Lectures on Riemann surfaces (Vol. 81). Springer Science & Business Media.
  119. ^ Miranda, R. (1995). Algebraic curves and Riemann surfaces (Vol. 5). American Mathematical Soc.
  120. ^ Donaldson, S. (2011). Riemann surfaces. Oxford University Press.
  121. ^ Serre, J. P. (1955). Faisceaux algébriques cohérents. Annals of Mathematics, 197–278.
  122. ^ Serre, J. P. (1956). Géométrie algébrique et géométrie analytique. In Annales de l'Institut Fourier (vol. 6, pp. 1–42).
  123. ^ Jiří Matoušek (2013). Lectures on Discrete Geometry. Springer Science & Business Media. ISBN 978-1-4613-0039-7.
  124. ^ Chuanming Zong (2006). The Cube – A Window to Convex and Discrete Geometry. Cambridge University Press. ISBN 978-0-521-85535-8.
  125. ^ Peter M. Gruber (2007). Convex and Discrete Geometry. Springer Science & Business Media. ISBN 978-3-540-71133-9.
  126. ^ Satyan L. Devadoss; Joseph O'Rourke (2011). Discrete and Computational Geometry. Princeton University Press. ISBN 978-1-4008-3898-1.
  127. ^ Károly Bezdek (2010). Classical Topics in Discrete Geometry. Springer Science & Business Media. ISBN 978-1-4419-0600-7.
  128. ^ Franco P. Preparata; Michael I. Shamos (2012). Computational Geometry: An Introduction. Springer Science & Business Media. ISBN 978-1-4612-1098-6.
  129. ^ Xianfeng David Gu; Shing-Tung Yau (2008). Computational Conformal Geometry. International Press. ISBN 978-1-57146-171-1.
  130. ^ a b Clara Löh (2017). Geometric Group Theory: An Introduction. Springer. ISBN 978-3-319-72254-2.
  131. ^ John Morgan; Gang Tian (2014). The Geometrization Conjecture. American Mathematical Soc. ISBN 978-0-8218-5201-9.
  132. ^ Daniel T. Wise (2012). From Riches to Raags: 3-Manifolds, Right-Angled Artin Groups, and Cubical Geometry: 3-manifolds, Right-angled Artin Groups, and Cubical Geometry. American Mathematical Soc. ISBN 978-0-8218-8800-1.
  133. ^ a b Gerard Meurant (2014). Handbook of Convex Geometry. Elsevier Science. ISBN 978-0-08-093439-6.
  134. ^ Jürgen Richter-Gebert (2011). Perspectives on Projective Geometry: A Guided Tour Through Real and Complex Geometry. Springer Science & Business Media. ISBN 978-3-642-17286-1.
  135. ^ Kimberly Elam (2001). Geometry of Design: Studies in Proportion and Composition. Princeton Architectural Press. ISBN 978-1-56898-249-6.
  136. ^ Brad J. Guigar (2004). The Everything Cartooning Book: Create Unique And Inspired Cartoons For Fun And Profit. Adams Media. pp. 82–. ISBN 978-1-4405-2305-2.
  137. ^ Mario Livio (2008). The Golden Ratio: The Story of PHI, the World's Most Astonishing Number. Crown/Archetype. p. 166. ISBN 978-0-307-48552-6.
  138. ^ Michele Emmer; Doris Schattschneider (2007). M. C. Escher's Legacy: A Centennial Celebration. Springer. p. 107. ISBN 978-3-540-28849-7.
  139. ^ Robert Capitolo; Ken Schwab (2004). Drawing Course 101. Sterling Publishing Company, Inc. p. 22. ISBN 978-1-4027-0383-6.
  140. ^ Phyllis Gelineau (2011). Integrating the Arts Across the Elementary School Curriculum. Cengage Learning. p. 55. ISBN 978-1-111-30126-2.
  141. ^ Cristiano Ceccato; Lars Hesselgren; Mark Pauly; Helmut Pottmann, Johannes Wallner (2016). Advances in Architectural Geometry 2010. Birkhäuser. p. 6. ISBN 978-3-99043-371-3.
  142. ^ Helmut Pottmann (2007). Architectural geometry. Bentley Institute Press. ISBN 978-1-934493-04-5.
  143. ^ Marian Moffett; Michael W. Fazio; Lawrence Wodehouse (2003). A World History of Architecture. Laurence King Publishing. p. 371. ISBN 978-1-85669-371-4.
  144. ^ Robin M. Green; Robin Michael Green (1985). Spherical Astronomy. Cambridge University Press. p. 1. ISBN 978-0-521-31779-5.
  145. ^ Dmitriĭ Vladimirovich Alekseevskiĭ (2008). Recent Developments in Pseudo-Riemannian Geometry. European Mathematical Society. ISBN 978-3-03719-051-7.
  146. ^ Bengtsson, Ingemar; Życzkowski, Karol (2017). Geometry of Quantum States: An Introduction to Quantum Entanglement (2nd ed.). Cambridge University Press. ISBN 978-1-107-02625-4. OCLC 1004572791.
  147. ^ Shing-Tung Yau; Steve Nadis (2010). The Shape of Inner Space: String Theory and the Geometry of the Universe's Hidden Dimensions. Basic Books. ISBN 978-0-465-02266-3.
  148. ^ Harley Flanders; Justin J. Price (2014). Calculus with Analytic Geometry. Elsevier Science. ISBN 978-1-4832-6240-6.
  149. ^ Jon Rogawski; Colin Adams (2015). Calculus. W. H. Freeman. ISBN 978-1-4641-7499-5.
  150. ^ Álvaro Lozano-Robledo (2019). Number Theory and Geometry: An Introduction to Arithmetic Geometry. American Mathematical Soc. ISBN 978-1-4704-5016-8.
  151. ^ Arturo Sangalli (2009). Pythagoras' Revenge: A Mathematical Mystery. Princeton University Press. p. 57. ISBN 978-0-691-04955-7.
  152. ^ Gary Cornell; Joseph H. Silverman; Glenn Stevens (2013). Modular Forms and Fermat's Last Theorem. Springer Science & Business Media. ISBN 978-1-4612-1974-3.

Sources

Further reading

External links

Wikibooks has more on the topic of: Geometry
Library resources about
Geometry

"Geometry" . Encyclopædia Britannica. Vol. 11 (11th ed.). 1911. pp. 675–736.

Retrieved from "https://dawoum.duckdns.org/w/index.php?title=Geometry&oldid=82268"